Question
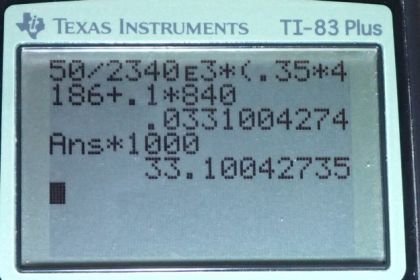
How many grams of coffee must evaporate from 350 g of coffee in a 100-g glass cup to cool the coffee from to ? You may assume the coffee has the same thermal properties as water and that the average heat of vaporization is 2340 kJ/kg (560 cal/g). (You may neglect the change in mass of the coffee as it cools, which will give you an answer that is slightly larger than correct.)
Final Answer
Solution video
OpenStax College Physics, Chapter 14, Problem 19 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a 100 grams glass coffee cup containing 350 grams of coffee and its going to cool from 95 degree Celsius to 45 degree Celsius. And so we convert these masses in grams into kilograms by dividing by 1000 and we change this 95 and 45 into temperature difference because that is what we use in our heat formula and that change of temperature is 50 Celsius degrees and we have some coffee evaporating and latent heat of vaporization is going to be 2340 times ten to the three joules per kilogram. Now this is not the latent heat of vaporization that you normally have in for water. Its not the value you find in table 14.2 because the water is not 100 degree Celsius. So, this is you know just that assumption but its gonna be something less than that because of its lower temperature. So, I have also written down the specific heat of water and glass and… and you know I could have written c for coffee here, but we were told coffee has the thermal properties of the water. So, we have the latent heat of the vaporization that’s going to be transfer to weight due to the evaporation is gonna be equal to the change of temperature of the water plus the change of heat due to de causing the change of temperature of the glass coffee cup and so that’s the mass that evaporates times latent heat of vaporization equals the mass of water times the specific heat of water times the change of temperature of water and I did not put the subscript w on this delta T because the water’s temperature and the glass temperature will be changing by the same amount so there is no need to distinguish those factors and we are gonna add to this the by the heat transfer out of the glass which is the mass of the glass times specific heat of the glass times change of the temperature. Let me factor out that common factor delta T here and then we solve for m the amount of coffee that has to evaporate by dividing both sides by latent heat of vaporization and then we get this formula here. So, the mass is going to be tangent temperature which is 50 Celsius degrees divided by latent heat of vaporization at this particular temperature and that’s gonna be 2340 times ten to the three joules per kilogram times .35 kilogram of coffee times specific heat of coffee which is that of water plus the mass of the glass which is 0.1 kilogram times 840 joules per kilogram per Celsius degree specific heat for glass and we get an answer in kilograms which we convert by into grams and 33.1 grams of coffee must evaporate to change temperature.