Question
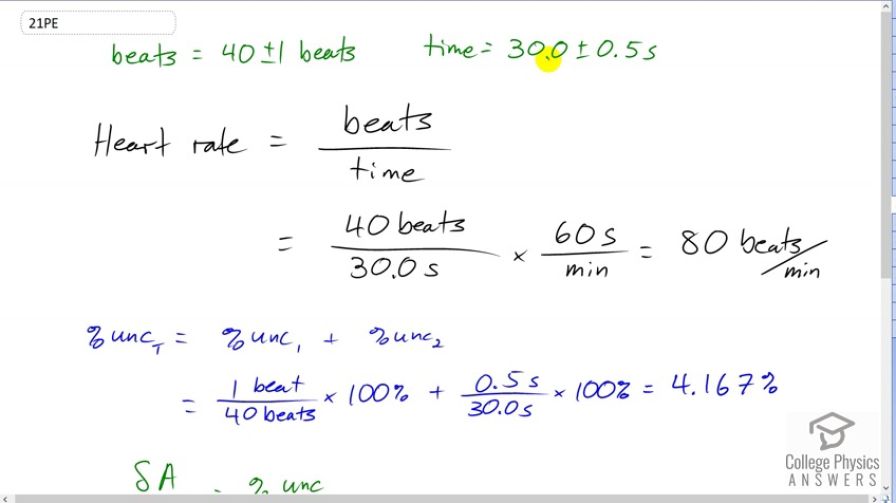
A person measures his or her heart rate by counting the number of beats in . If beats are counted in
, what is the heart rate and its uncertainty in beats per minute?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 1, Problem 21 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko The data we're given in this question is that there are 40 plus or minus 1 beats measured in a time of 30.0 plus or minus 0.5 seconds. And so, our job is to figure out what the heart rate is, which we’ll calculate by dividing beats by time and then also include what the uncertainty is in that result. So, we have 40 beats divided by 30.0 seconds and we can do this calculation of the heart rate without thinking about the uncertainties because we're going to think about those in the next step. And so, we have 40 divided by 30. It's not quite good enough because the answer tells us to... or the question tells us to express our heart rate in beats per minute. So, we need to multiply it by a conversion factor so that we get rid of seconds and be left with minutes. And there are 60 seconds per minute and we're left with 80 beats per minute. It's our heart rate. Now, for the uncertainty calculation, since we are doing dividing, we are going to add percent uncertainties in the numbers that we’re dividing in order to find the percent uncertainty in our answer. I put a subscript capital T here for the total. The total percent uncertainty in a calculation involving dividing or multiplying is the sum of the percent uncertainties in the first number, in the second number and so on of all the numbers that you're multiplying or dividing. And so, we find the percent uncertainty in the beats measurement and that is one beat for every... divided by 40 beats and times by a 100%. And then, the percent uncertainty in the time is half a second divided by 30 seconds. And this gives us 4.167%, and we'll leave it as a number with lots of decimals just because it's an intermediate calculation. It's not a final answer and we're going to find the absolute uncertainty in the heart rate. And so, the textbook formula is Delta A over A equals percent uncertainty, delta A being the amount of uncertainty in absolute terms in the same units as the measurement, the denominator being the measurement. And then we’ll rearrange this by multiplying both sides by A and it cancels the left, leaving us with the uncertainty as percent uncertainty times the measurement. So, the uncertainty in the heart rate which are abbreviated capital H, capital R equals the percent uncertainty that we calculated multiplied by the measurement or the result of our calculation anyhow of 80 beats per minute and that gives 3.333 beats per minute. It is our uncertainty. And we will round that to the ones place because this uncertainty is a number that we will add or subtract to the measurement and we’ll assume that this 80 has two significant figures. We’ll assume that the ones place is significant although it's a bit hard to tell because... actually it's not hard to tell. No, it definitely is significant to the ones place because our uncertainty is in the ones place. So, this zero is significant. So, when adding or subtracting, your answer can be only as precise as the least precise number. And so, this one, well, we're not sure how precise it should be. Maybe, it's precise to the tens place. It's hard to tell, but when we're going to add it or subtract it to this number 80, we know that this sum or difference can be only as precise as the ones place, since 80 is limited by this precision here. And so, it makes sense to have only the ones place in our uncertainty as well. There. So, the answer is our heart rate is 80 plus or minus 3 beats per minute.
