OpenStax College Physics for AP® Courses, Chapter 9, Problem 4 (Problems & Exercises)

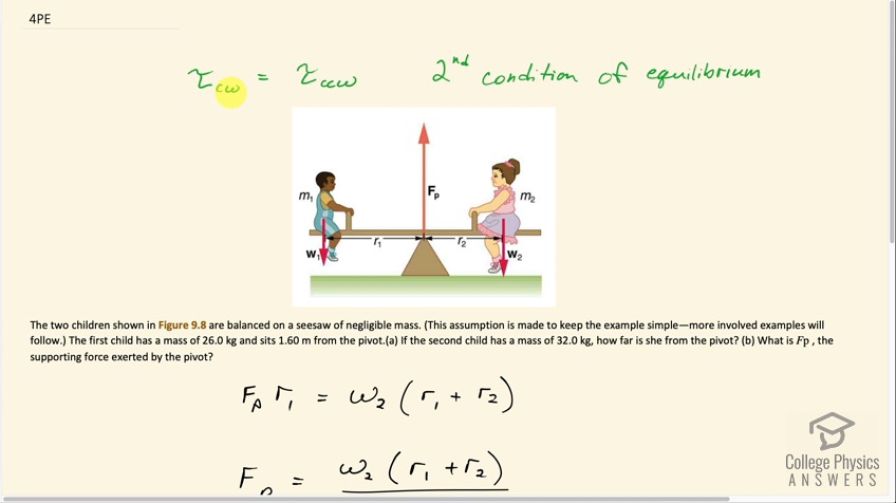
This is College Physics Answers with Shaun Dychko. We are going to calculate the force exerted by the pivot using the second condition of equilibrium which is to say that the total clockwise torques have to equal the total counter-clockwise torques. So the pivot point exerts a torque that is counter-clockwise about a pivot located here and you can choose your pivot wherever you want it to be, it doesn't have to be... I mean the fulcrum seems to be the most obvious place to choose the pivot but we can't choose it there and figure out the force exerted by the pivot since it would have zero torque about a pivot that is zero meters away. So we have to choose a pivot in a different position in order to use a second condition of equilibrium here for this force. So this pivot point is exerting a counter-clockwise torque a distance r 1 from this pivot and that's going to equal the clockwise torque exerted by child number 2 which is a weight distance r 1 plus r 2 from this pivot. So we'll divide both sides by r 1 and solve for the force exerted by the pivot and then substitute in mass 2 times g in place of the weight of child 2. And that's 32.0 kilograms times 9.80 newtons per kilogram times 1.60 meters plus 1.30 meters and this 1.30 meters—the distance of child 2 from the pivot— was solved for in the example and so we'll use that result here and we divide by 1.60 and we get 568 newtons.