Question
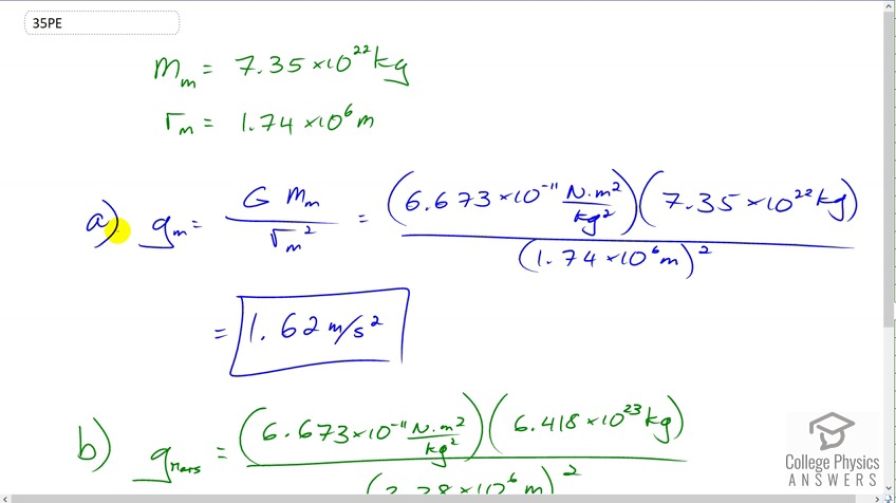
(a) What is the acceleration due to gravity on the surface of the Moon?
(b) On the surface of Mars? The mass of Mars is and its radius is 3.38 \times 10^6 \textrm{ m} .
Final Answer
a)
b)
Solution video
OpenStax College Physics for AP® Courses, Chapter 6, Problem 35 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Acceleration due to gravity equals the universal gravitational constant multiplied by the mass of the body, the moon in part A or Mars in part B, divided by the radius of the body. So for the moon, acceleration due to gravity is going to be 6.673 times ten to the minus eleven multiplied by the mass of the moon, 7.35 times ten to the twenty-two kilograms which is some data that I looked up in the back of the text book in the Appendix, and divided by 1.74 times ten to the six meters squared, giving 1.62 meters per second squared as the acceleration due to gravity on the moon. Then on the surface of Mars, we have the gravitational constant multiplied by the mass of Mars, which is 6.418 times ten to the twenty-three kilograms, divided by the average radius of Mars which is 3.38 times ten to the six meters squared, giving an acceleration due to gravity on the surface of Mars of 3.75 meters per second squared.
