Question
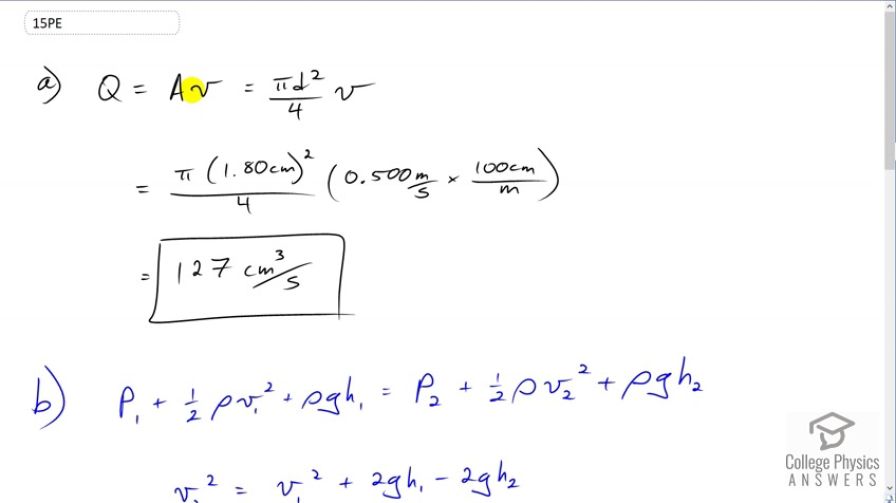
Water emerges straight down from a faucet with a 1.80-cm diameter at a speed of 0.500 m/s. (Because of the construction of the faucet, there is no variation in speed across the stream.) (a) What is the flow rate in ? (b) What is the diameter of the stream 0.200 m below the faucet?
Neglect any effects due to surface tension.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 12, Problem 15 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We can figure out the volume flow rate of this water from the faucet by saying that it's equal to the cross-sectional area of the faucet multiplied by the speed of the water coming out of it. So the cross-sectional area, we can figure out from the diameter we're given by going Pi times diameter squared over four and then multiply that by the speed that we're also given. So that's Pi times 1.8 centimeters squared over four times 0.5 meters per second which we have to convert into centimeters in order to be consistent with the centimeters that we've used over here. And so 0.5 times 100 centimeters for every meter and we end up with 127 cubic centimeters per second. And then, we're told that the water falls some distances head to the faucet. So here is the mouth of the faucet right there. The water will flow out and the diameter of the stream will get smaller as it falls downwards. So here's 0.2 meters and the question is, what is the diameter of the stream here? So we'll call this d2. And this up here is d1. And we'll use Bernoulli's Equation in order to figure this out. So, Bernoulli's Equation says that the pressure at an initial location in a stream of incompressible fluid is plus one half times a fluid's density times its initial speed squared plus its density times g times initial height h1 equals the pressure kinetic energy and gravitational potential energy at some other position. Now, the pressure at the initial and final position in these two cases are the same because at the mouth of the faucet, the pressure is atmospheric pressure. And likewise down here, it's also atmospheric pressure. And so P1 and P2 are equal and so they cancel. And now we're left with four terms all containing with the same factor density so that also cancels. And we can solve for V2 squared because we want to figure out what the speed of the fluid is at this point, because if we know the speed and we can assume that the volume flow rate will be the same down here as it is up here. And then if we know the speed, we can figure out the cross-sectional area and therefore, the diameter. So, V2 squared is V1 squared plus two gh1 minus two gh2. So I did a couple of things there. First I moved this term to the left side to make it minus gh2 and then I multiplied everything by two to get rid of this factor one half in front of the V2 squared. So that's why there's a two in front of both of these terms and this have factor one half to begin with. And so multiplying it by two just made a factor of one in front of it. So, we have V2 squared as V1 squared plus two g times h1 minus h2. And this is the change in height which we're given and we're told that it falls 0.2 meters. So V2 is going to be the square root of all that. And so we have square root of 0.5 meters per second squared plus two times 9.81 meters per second squared times the change in height of 0.2 meters. And this gives 2.04304 meters per second. And we expect that to be faster than we had before in part a, at the mouth of the faucet with 0.5 meters per second. And now we're up to 2.04 meters per second. So that makes sense. Now the volume flow rate at this position lower down Q2 is going to be the same as the volume flow rate at the mouth of the faucet Q1. And the volume flow rate also can be noted as the cross-sectional area with a subscript two there because this is a new cross-sectional area, because it is a new diameter of the fluid column times V2. And that is Pi times diameter two squared over four times V2 and all of this equals Q1. And then we'll solve for d2 squared by multiplying both sides by four over Pi V2. And we get d2 squared is four Q1 over PiV2. And then take the square root of both sides and you get d2 as two times square root Q1 over Pi V2. So that's two times square root of 127.235 cubic centimeters per second and then divided by Pi times the speed that we calculated up here, 2.04304 meters per second. But be careful with the units. They have to be consistent. So this speed unit at the bottom should be converted into centimeters per second to be consistent with the cubic centimeters per second in the numerator. And this all works out to 0.890 centimeters. And to do a bit of a reality check on that number, we expect from our experience that the faucet gets more or the stream of water from a faucet gets more narrow as the stream falls. And so we expect this diameter 0.89 centimeters to be smaller than the diameter of the faucet and sure enough it is, it started as 1.8 centimeters before at the faucet mouth.

