Question
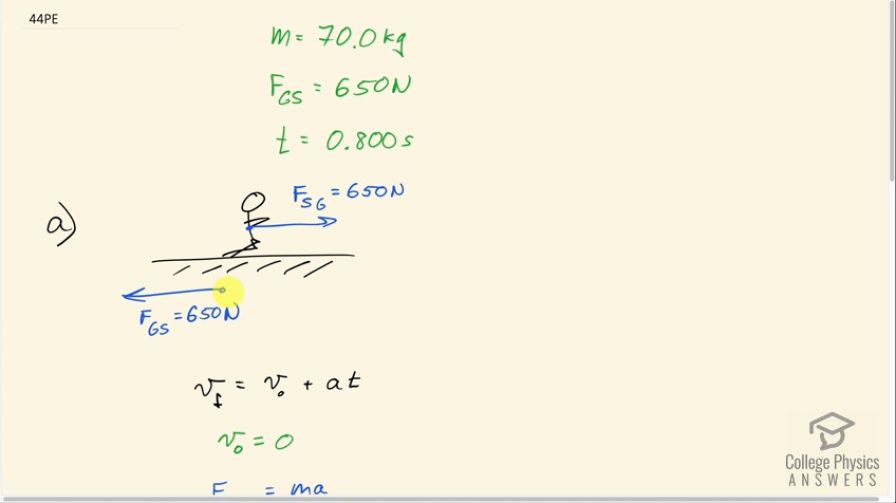
Integrated Concepts When starting a foot race, a 70.0-kg sprinter exerts an average force of 650 N backward on the ground for 0.800 s. (a) What is his final speed? (b) How far does he travel?
Final Answer
Solution video
OpenStax College Physics, Chapter 4, Problem 44 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The sprinter is exerting a force on the ground backwards of 650 newtons and the ground will, in turn, exert a Newton's third law counterpart on the sprinter of an equal magnitude force in the opposite direction. The sprinter experiences a force due to the ground of 650 newtons forwards. The time that this force is applied is point eight seconds and the sprinter's mass at 70 kilograms, and we have to answer and part A what is the final speed going to be. We know the final speed is initial speed plus acceleration times time. The initial speed we take to be zero. The acceleration we can figure out from Newton's second law that the net force equals mass times acceleration. We divide both sides by m to solve for a after switching the sides around and we get the acceleration that is the net force divided by m. We can see that only one force is acting horizontally on the sprinter because we're neglecting air friction of course. That means that force is the force on the sprinter due to the ground. We substitute that in. This acceleration gets substituted into our kinematics formula here in place of a. We have that the final speed then is zero initial speed, plus the force divided by the mass times time. So 650 newtons divided by 70 kilograms times 0.8 seconds, which is 7.43 meters per second. Part B asked us how far the sprinter will go during this 0.8 seconds of acceleration. We know that the final position will be the initial position plus the initial velocity multiplied by time plus one half times acceleration times time squared. The initial position will take to be zero. The initial speed we know is zero, so this whole term is zero. Then we have substituting the force divided by mass in place of acceleration just as we did in part A. We have them at the final position then or the distance traveled, in other words, is one-half times 650 newtons divided by 70 kilograms times 0.8 seconds squared which is 2.97 meters.
