Question
The weight of an astronaut plus his space suit on the Moon is only 250 N. How much do they weigh on Earth? What is the mass on the Moon? On Earth?
Final Answer
,
Solution video
OpenStax College Physics, Chapter 4, Problem 13 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
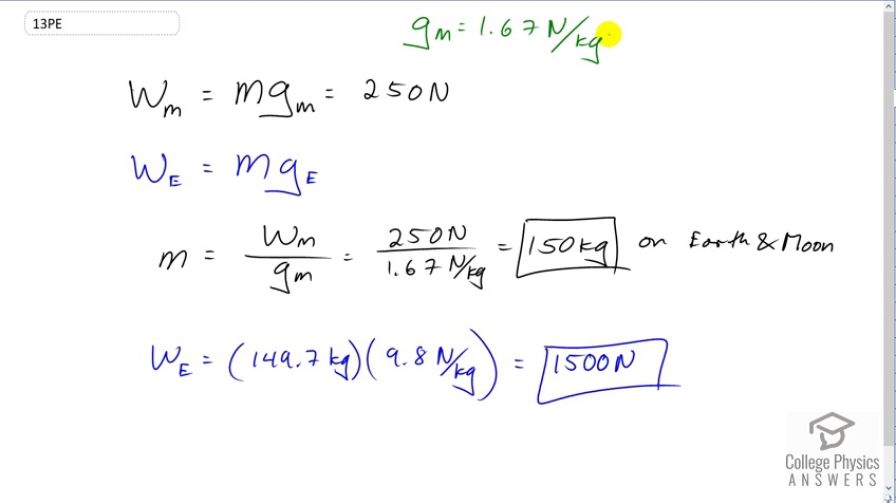
This is College Physics Answers with Shaun Dychko. We have to look up the gravitational field strength of the moon and it's 1.67 newtons per kilogram. Often you'll see these units written as meters per second squared which is also totally fine, but newtons per kilogram is another way to write it and it'd be a good exercise if you wanted to verify that that's true. But it's actually not very hard for me to do just right now. newtons comes from mass times acceleration so kilograms times meters per second squared, and so if you're dividing this newtons by kilograms, you'll see that the kilograms cancel leaving us with meters per second squared. So it's an alternative way of writing the same thing and in this case, it makes more sense to me anyway because we have newtons divided by newtons per kilogram and this is going to work out to kilograms. So, we have weight on the moon is the mass of the astronaut times the moon's gravitational field strength, that we're told is 250 newtons. Weight on earth will be the same mass because the mass doesn't change only the weight does, and multiply by the gravitational field strength of the earth. Return to the moon situation because we have some known values there we can use to calculate mass. So the weight divided by g, and we can divide both sides of this equation by gm and switch the sides around. You get the mass, 250 newtons divided by 1.67 newtons per kilogram, is 150 kilograms on earth and on the moon. So the weight on earth will be that mass which I've written with more significant figures here in order to -- well, it's not more significant figures, it's more digits. It has only two significant figures because this number has only two significant figures, but I've written lots of digits here to avoid intermediate rounding error. So I have 149.7 kilograms, times 9.8 newtons per kilogram gravitational field strength of earth and this works out to 1500 newtons, also which I have rounded to two significant figures.
