Question
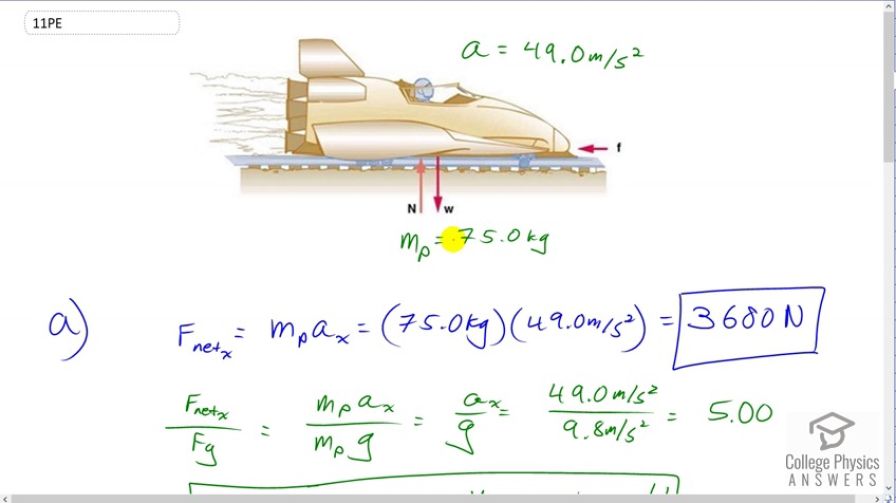
The rocket sled shown in Figure 4.32 accelerates at a rate of . Its passenger has a mass of 75.0 kg. (a) Calculate the horizontal component of the force the seat exerts against his body. Compare this with his weight by using a ratio. (b) Calculate the direction and magnitude of the total force the seat exerts against his body.
Final Answer
a) . It is times the passenger's weight.
b)
Solution video
OpenStax College Physics, Chapter 4, Problem 11 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
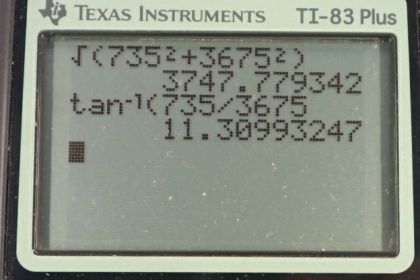
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The passenger in this rocket sled is going to be accelerating at 49 meters per second squared and we're told that their mass is 75 kilograms. So we can figure out the x component of the net force being exerted on the passenger by multiplying their mass by their acceleration in the x direction. That is 75 kilograms, times 49 meters per second squared which gives 3680 newtons. Then we're asked to find what is that compared to their weight. The mass of the person times the x component of their acceleration, divided by mass of the person times g, this being the weight, force of gravity, and the masses are going to cancel, and so really we're taking the ratio of the accelerations and this works out to 5. So the horizontal force exerted by the seat which is also the net force x component, is five times the person's weight. Here's a picture of the passenger on the seat and the three forces on them, one being horizontal. Then there is the normal force exerted upwards by the seat and the force of gravity down. This red arrow is going to be the net force exerted by the seat. So, the picture might be a little bit confusing because this is not the resultant force of all three. Instead it is only considering these two because these are the two forces exerted by the seat. So, vertically speaking the normal force minus the force of gravity is zero because the passenger is not accelerating vertically and so we can add fg to both sides. We get normal forces equal to the weight which is mg and 75 times 9.8, that gives 735 newtons. So the vector is riding together to get this resultant force exerted by the seat is the normal force plus the x component of the net force. So these are perpendicular which is convenient, and so we can find the magnitude of this resultant force by taking the square root of each of them squared added together. This is 735 newtons squared plus 3675 newtons squared, and that gives 3750 newtons. The angle will be the vertical component divided by the horizontal component of this resultant force and then take the inverse tangent of that. So that's the inverse tangent of 735 over 3675, giving us 11.3 degrees. So the final answer for the resultant force exerted by the seat on the passenger is 3750 newtons, 11.3 degrees above horizontal.