Question
Plans for an accelerator that produces a secondary beam of K-mesons to scatter from nuclei, for the purpose of studying the strong force, call for them to have a kinetic energy of 500 MeV. (a) What would the relativistic quantity be for these particles? (b) How long would their average lifetime be in the laboratory? (c) How far could they travel in this time?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 33, Problem 48 (Problems & Exercises)
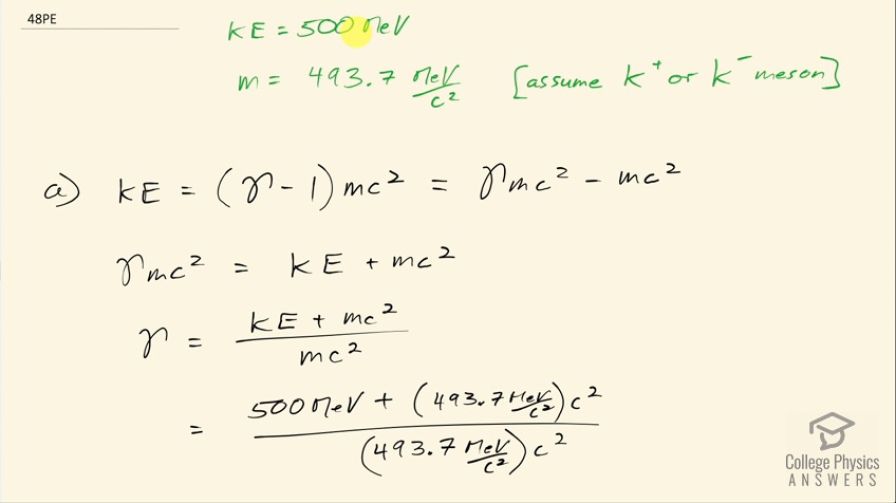
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We suppose that an accelerator is producing a beam of K mesons that have a kinetic energy of 500 megaelectron volts. We need to know what the mass of these mesons are so we look at table [33.2] and we see that there are two types of K mesons and the question isn't clear about which type this accelerator is creating so we just have to make an assumption. These ones are I guess easier to create because they have less mass so let's assume that it's either a K plus or a K minus in which case, its mass is 493.7 megaelectron volts per c squared. Question (a) is asking what would gamma be, this Lorentz factor? Well we know that kinetic energy is gamma minus 1 times the rest mass times c squared and we can expand this bracket by multiplying both terms by mc squared and we get γmc squared minus mc squared. Then we add mc squared to both sides and we get γmc squared equals kinetic energy plus mc squared then divide both sides by mc squared and then we get γ. So γ is the kinetic energy 500 megaelectron volts plus the rest mass 493.7 megaelectron volts per c squared times c squared divided by the rest mass times c squared and we get 2.01 is γ. And then the question is asking what will the lifetime be in the laboratory for this particle? Well its lifetime will be this Lorentz factor γ multiplied by the lifetime that the particle has in its own reference frame or in other words, the proper time. So we need to look up that proper time and that's shown in this column here— 1.24 times 10 to the minus 8 seconds— is a lifetime of the particle when it's at rest. So we multiply that lifetime by Lorentz factor and we get 2.50 times 10 to the minus 8 seconds is the lifetime as observed by a person in a laboratory watching the particle zip by. Part (c) is asking how far could this particle travel? Well we need to know what its speed is so we will need to solve this expression for γ and solve it for v. So we can square both sides and we have gamma squared then is 1 over 1 minus the speed of the particle squared over c squared. Then we multiply both sides by 1 minus v squared over c squared and also divide both sides by γ squared and we get 1 minus v squared over c squared equals 1 over γ squared. And then we'll add v squared over c squared to both sides and then also subtract 1 over γ squared from both sides and switch the sides around and we get v squared over c squared equals 1 minus 1 over γ squared. Then multiply both sides by c squared and we get v squared equals c squared times 1 minus 1 over γ squared and then take the square root of both sides and we get v equals c times square root 1 minus 1 over γ squared. So that's 2.998 times 10 to the 8 meters per second times square root of 1 minus 1 over 2.0128 squared and that's 2.6018 times 10 to the 8 meters per second. So we multiply that speed by the lifetime of the particle in the reference frame of the laboratory and we get a distance that a person in the lab will measure of 6.49 meters.