Question
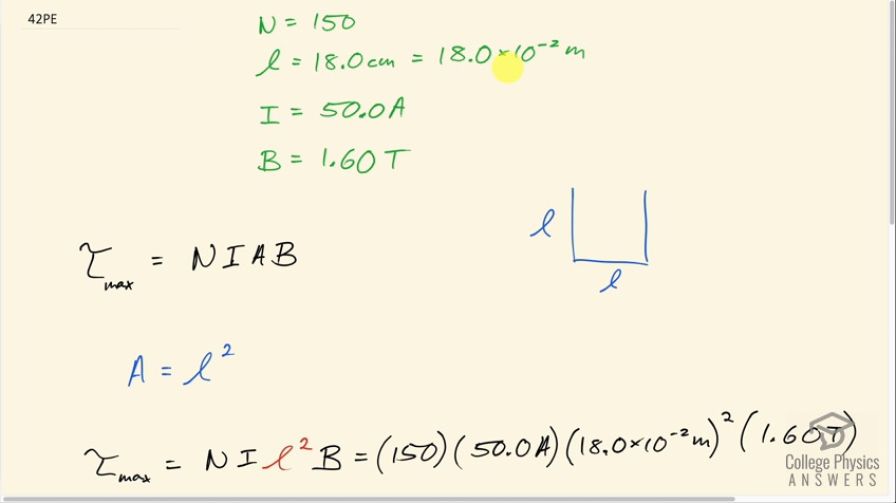
(a) What is the maximum torque on a 150-turn square loop of wire 18.0 cm on a side that carries a 50.0-A current in a 1.60-T field? (b) What is the torque when is ?
Final Answer
- 389 \textrm{ N}\cdot\textrm{m}
Solution video
OpenStax College Physics for AP® Courses, Chapter 22, Problem 42 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A square loop that has 150 turns is 18.0 centimeters on a side and that is 18.0 times 10 to the minus 2 meters and it carries 50.0 amps in a magnetic field of 1.60 tesla and the loop is oriented such that the torque is at a maximum right now. So in this picture, the field lines might be like this say and the current might be, you know, down like this, up like that. And to calculate that maximum torque, we take the number of turns multiply it by the current, multiply it by the area of the loop multiply it by the magnetic field strength. So the area is gonna be the side multiplied by the other side and they are the same so it's gonna be l squared— the length of one side squared because it's a square. So we substitute that in for A and we do that here and so the maximum torque then is 150 turns times 50.0 amps times 18.0 times 10 to the minus 2 meters squared times 1.60 tesla, which is 389 newton meters. Now in this part (b), we are told everything is the same except the angle is now 10.9 degrees so we have to multiply by this sin Θ which I didn't bother writing up here; I could have written multiplied by sin of 90 that would give the same answer because sin of 90 is 1. Now in this case here, we have sin of 10.9 degrees multiplied by everything else as we did before in part (a) and we get 73.5 newton meters for the torque.
