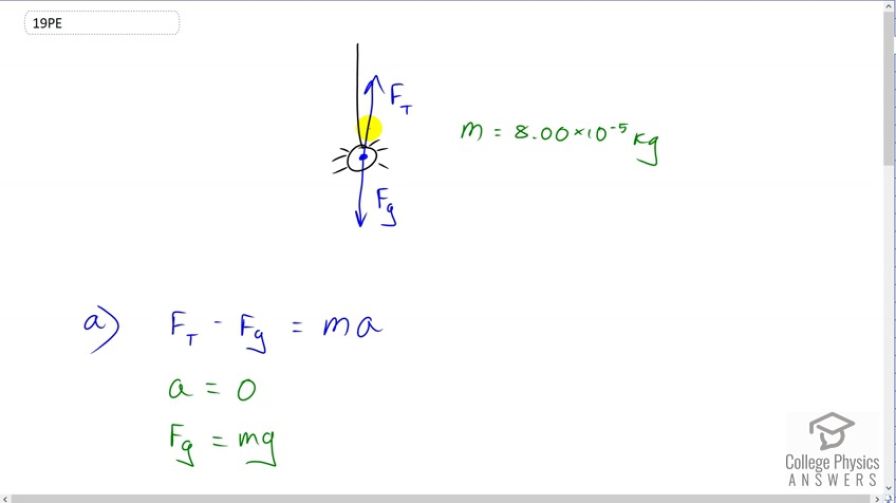
a)
b) , which is 2.4 times the tension in the vertical strand.
Note: The video didn't show finding the ratio of the answer to part (b) vs part (a), but this calculation is shown in the second calculator screenshot where the answer to part (b) is divided by the answer to part (a) to get the result of 2.4.Solution video
OpenStax College Physics, Chapter 4, Problem 19 (Problems & Exercises)
Calculator Screenshots
Comments
This instructor is going way to fast, will be not be a subscriber anymore.
Why do we only consider the y component for part b)?
Thank you for the question. The y-component of the tension in each segment of the wire is the only force exerted by the wire on the spider. The horizontal components of the tension are not exerted on the spider, so they have no place in the net Force calculations. Strictly speaking, part (b) does not show a free body diagram of the spider, which perhaps makes it confusing. A free body diagram of the spider would have one arrow down representing gravity, and one arrow of equal length pointing up representing the total of the y-components of the tension in each segment of wire. This means only the y-components appear in our calculations.
Hope this helps,
Shaun
Can you explain what the x-components of the wire?
Why the answer only considers tension in 1/2 of the string, not the string as a whole?
Hi pansapinsa,
Thank you for the question. I think what you mean is why do we consider each half of the string separately? Since we add the y-component of the force due to each half together, we do get the total y-component of force on the spider. The approach of considering each half separately is necessary since we want to know the total force on the point where the spider is positioned, which is the middle. Tension in a stationary string is pulling equally in both directions. Since the strand isn't moving we know the net force is zero. The tension at that point is pulling both up-to-the-right and up-to-the-left, so we need to find the y-component of each of those forces.
Hope this helps a bit,
Shaun
L
Can you explain how you get that the y component is 2.4 times the x component.
Hello donahue, thank you for your comment. I have updated the final answer with this note: "The video didn't show finding the ratio of the answer to part (b) vs part (a), but this calculation is shown in the second calculator screenshot where the answer to part (b) is divided by the answer to part (a) to get the result of 2.4."
Thanks again, and all the best,
Shaun
Why do you put 2/sin(12) in the calculator when you say the formula has 2 multiplied by sin(12)?
Hi tyler, thank you for your question. This has to do with a personal preference on how to enter things into the calculator. I think what you're expecting to see, and rightly so, is in the denominator of the calculator screenshot. That would be correct. What I've done instead, which is also correct, is to save the hassle of typing brackets surrounding the denominator by dividing by each factor one after the other. As an example to explain, the following two calculations give the same result: (your way: ) and (my way:) . The calculator goes from left to right in my example when given operations of equal precedence (division in this case) and evaluates the first before proceeding to the second. to put it more explicitly.
Hope this helps,
Shaun




