OpenStax College Physics for AP® Courses, Chapter 4, Problem 29 (Problems & Exercises)

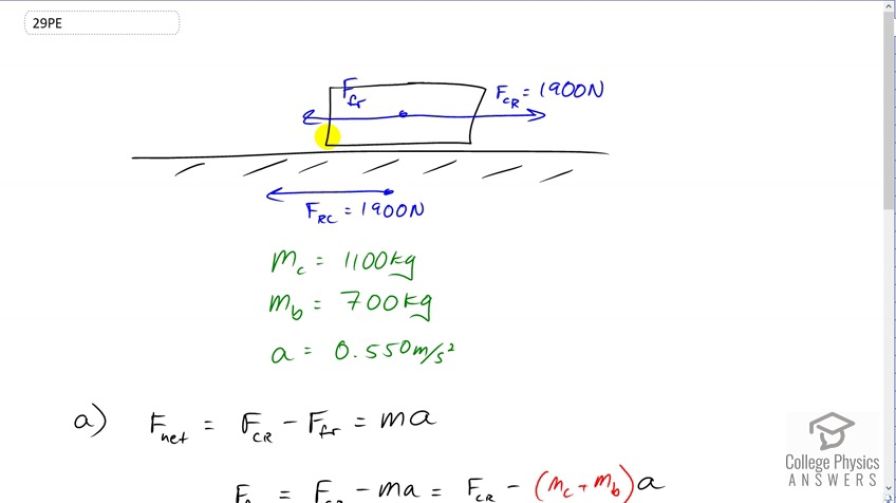
This is College Physics Answers with Shaun Dychko. We can think of this car and the boat and the trailer as all being one thing. So I've drawn just one black box here. We're told that the force exerted on the road by the car is 1900 newtons and that means that the force on the car due to the road is the same magnitude, but it's exerted in the opposite direction, to the right here. So this 1900 newtons is the force that will be exerted on this whole system, car, boat, and trailer. We're told there's some total -- no retarding or friction force as well on the system. We're going to find out what that is. So the mass of the car is 1100 kilograms and the mass of the boat and trailer together is 700 and the system accelerates at 0.55 meters per second squared. So we've drawn a free body diagram which is this here. This arrow here is not part of the free body diagram by the way, because the free body diagram is meant to draw just the system of interest which is the car and the boat and then show all the forces on that. This is the force on the ground. But I drew it anyway in order to explain where this force comes from. It's the Newton's third law counterpart to this one. Okay. So the net force horizontally is the force on the car due to the road, minus the total friction and that equals mass times acceleration. Then we'll add -- what do we do? We're going to add force of friction to both sides say, and then it becomes positive, and then minus m a from both sides and then switch the sides around. You end up with force of friction isolated and that equals the force on the car due to the road, minus mass times acceleration. Now the mass is the mass of the car plus the mass of the boat so I made a substitution for that here and then we plug in numbers. So the total force slowing down this whole system is 1900 newtons minus the mass of the car plus the mass of the boat and trailer, times 0.55 meters per second squared acceleration. That gives 910 newtons. Then in part B, we're told that suppose 80 percent of this friction is experienced by the boat and trailer. If that's the case, figure out what force must be exerted by the hitch on the trailer in order to make it accelerate the way it is. So I've drawn a free body diagram of the trailer and it has force of the hitch pointing to the right, and then it has the force of friction to the left which is 0.8 times the force of friction found in part A. This is force of friction within the posture piece so that's prime. So F fr prime is 0.8 times force of the friction. So the net force here is the hitch to the right minus the force of friction prime to the left and that equals the mass of the boat and trailer together, times acceleration. Acceleration does not have a prime nor a subscript because everything here is accelerating at the same rate. So the acceleration of the boat in part B is the same as the acceleration of this whole system in part A. So then, we add the friction to both sides and then we get force of the hitch is mass of the boat times acceleration, plus the force of friction on the boat and trailer. So it's 700 kilograms times 0.55 meters per second squared, plus 0.8 times 910 newtons that we figured out in part A being the total friction force of the system, and then we're taking 80 percent of that here. This works out to 1.11 times ten to the three newtons is the force exerted by the hitch on the trailer.