Question
(a) Calculate the tension in a vertical strand of spider web if a spider of mass hangs motionless on it. (b) Calculate the tension in a horizontal strand of spider web if the same spider sits motionless in the middle of it much like the tightrope walker in Figure 4.17. The strand sags at an angle of below the horizontal. Compare this with the tension in the vertical strand (find their ratio).
Final Answer
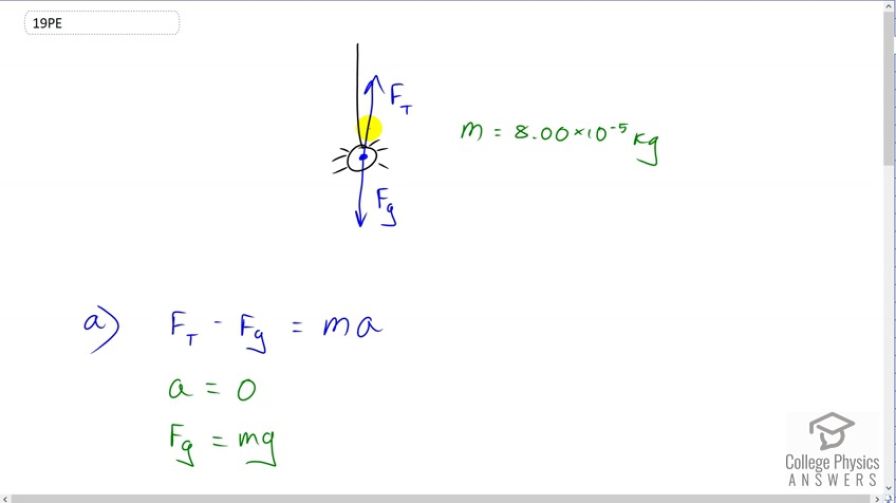
a)
b) , which is 2.4 times the tension in the vertical strand.
Note: The video didn't show finding the ratio of the answer to part (b) vs part (a), but this calculation is shown in the second calculator screenshot where the answer to part (b) is divided by the answer to part (a) to get the result of 2.4.Solution video
OpenStax College Physics for AP® Courses, Chapter 4, Problem 19 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This spider hanging from a vertical thread in part A has a mass of eight times ten to the minus five kilograms and our job is to find the tension in this spider silk thread. So the net force vertically is mass times acceleration and the net force is the tension upwards, minus the gravity downwards. We know that acceleration is zero because the spider is motionless we're told, and the force of gravity is the mass of the spider times the gravitational field strength g. So we can substitute for mg in place of Fg here and then well, since acceleration is zero, that means this term becomes zero. So add mg to both sides and you solve for force of tension and it is the weight in other words. So that's eight times ten to the minus five kilograms times 9.8 newtons per kilogram, giving 7.84 times ten to the minus four newtons. Now in part B, the spider is sitting in the middle of the horizontal section of the spider thread. We're told that the angle of depression of this thread is 12 degrees and that'll also be this angle here because these are -- these dotted lines are parallel lines and these are interior opposite angles, if you remember your parallel line rules for angles, and 12 degrees there as well. So this tension force is going to be exerted along the thread up into the right as well as along the thread up into the left and combined, these two tension forces are keeping the spider stationary. Now speaking about the vertical direction, the y direction, the net force will be two times the vertical component of each of these tension forces. Here is a vertical component of one of the tension forces here and it also exists on the other side so we have two of them supporting the spider, and that is the opposite leg of this triangle and so we use the sine function to find the opposite leg, multiplied by the hypotenuse which is Ft. So Ft y is sine theta times Ft. So, two times the vertical component of the tension upwards and then minus the weight downwards is the net force which is mass times acceleration. But acceleration is zero and so we can replace ma with zero. Fg is mg and so we replace that and then Ft y component is tension force times sine theta. So I replace that as well in this formula re-written here with a whole bunch of substitutions. Then we add mg to both sides, and then we have 2 Ftsine theta on the left and mg on the right. Then we'll solve for Ft by dividing both sides by two sine theta. So the tension force is the weight of the spider divided by two times the sine of the angle of depression of the thread. So that's 8 times ten to the minus five kilograms times 9.8, divided by two times sine of 12 which is 1.89 time ten to the minus three newtons.

