Question
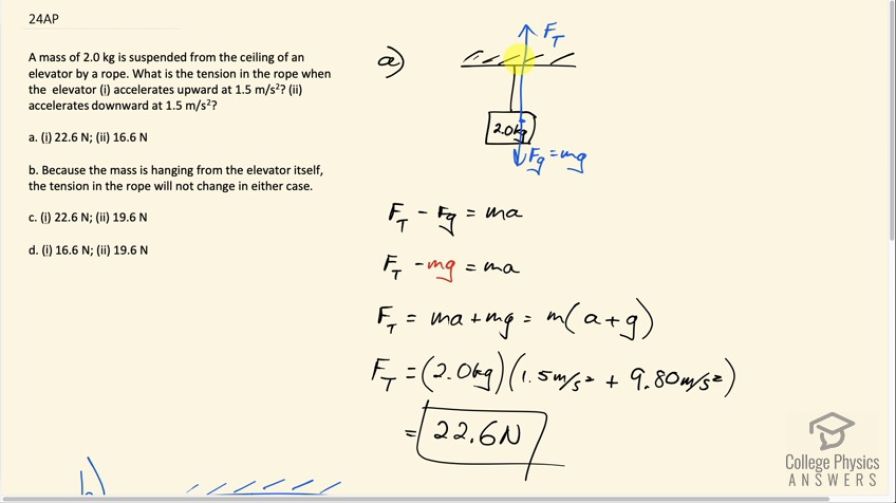
A mass of 2.0 kg is suspended from the ceiling of an elevator by a rope. What is the tension in the rope when the elevator (i) accelerates upward at ? (ii) accelerates downward at ?
- (i) 22.6 N; (ii) 16.6 N
- Because the mass is hanging from the elevator itself, the tension in the rope will not change in either case.
- (i) 22.6 N; (ii) 19.6 N
- (i) 16.6 N; (ii) 19.6 N
Final Answer
(a)
Solution video
OpenStax College Physics for AP® Courses, Chapter 4, Problem 24 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This two-kilogram mass is hanging from the ceiling of an elevator. The elevator is accelerating upwards at a rate of 1.5 meters per second square in part A. The tension force upwards must exceed the gravity downwards in order for this weight to be accelerating upwards. The tension up minus gravity down equals mass times acceleration, that's Newton's second law, and we replace F g with mg, and then add mg to both sides to solve for the tension force. That's ma plus mg and we factor out the common factor m and say m times a plus g. We have two kilograms times 1.5 meters per second squared acceleration upwards plus acceleration due to gravity 9.8 meters per second squared, getting a force of 22.6 newtons. This narrows it down to options A or C. We'll still have to figure out what happens in case two, when the elevator accelerates downwards at 1.5 meters per second squared. That picture is going to have the gravity vector down longer than the tension up. The form does the same though. But now we're going to have negative 1.5 meters per second squared in place of the acceleration. All this works out to 16.6 newtons of force upwards. So the answer is a.
