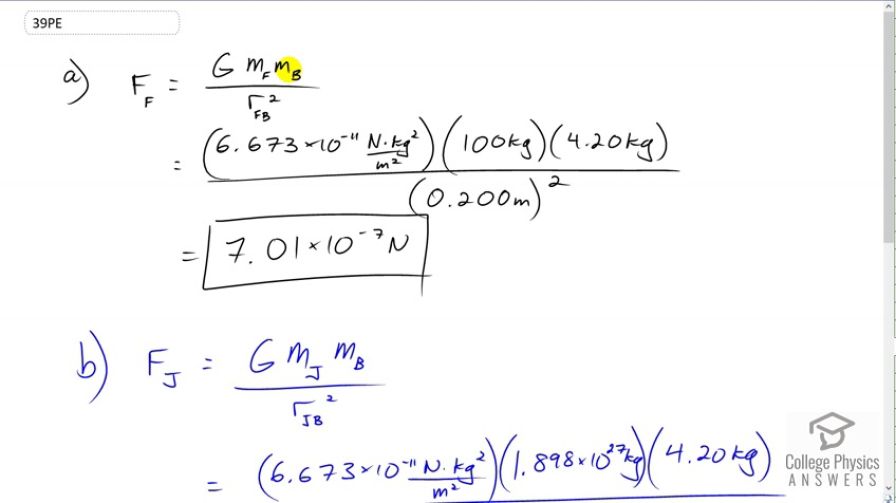
a)
b) . The force of gravity due to Jupiter is greater than that of the father by a factor of .
Solution video
OpenStax College Physics, Chapter 6, Problem 39 (Problems & Exercises)
Calculator Screenshots
Comments
In the video @ 45 seconds you have (1.898*10^27) but on the calculator you just do (1.898^27) Can you still get the correct answer doing it like that?
Hi bryanlovell, thanks for the question. With a closer look at the calculator screenshot, notice the capital "E" between 1.897 and 27. This is my favorite shorthand way of writing "times 10^". It has an advantage over typing "* 10^27" since the calculator understands that 1.897E27 should be treated as a single number. This means that when dividing by 1.897E27, the 10^27 factor stays in the denominator where it belongs with the 1.897 factor. To explain by example, for the problem , typing "20 / 1E1 = 2" which is correct, whereas "20 / 1 * 10^1 = 200" which is not the expected answer since the capital E wasn't used. Of course, one could type "20 / (1 * 10^1) = 2" to get the correct answer with brackets, but avoiding the trouble of typing those brackets is the whole point of the capital E shortcut.
I hope you enjoy the solutions,
Shaun



