Question
A mountain 10.0 km from a person exerts a gravitational force on him equal to 2.00% of his weight.
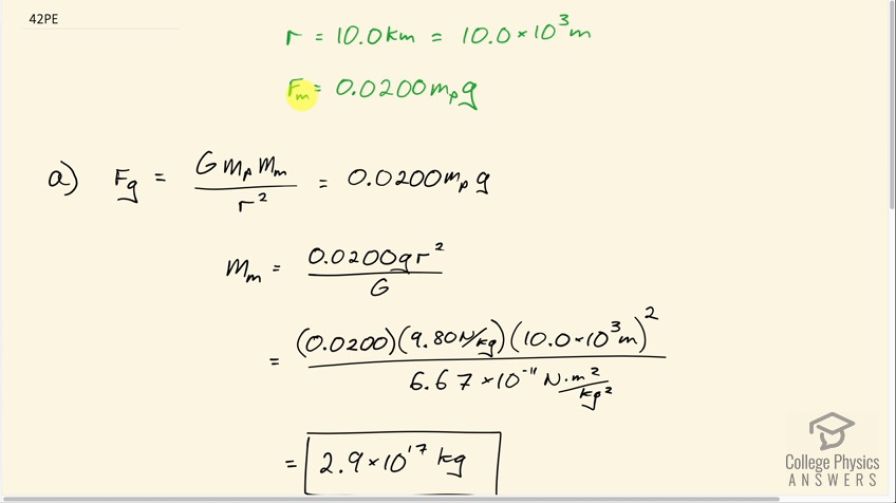
(a) Calculate the mass of the mountain.
(b) Compare the mountain's mass with that of Earth. (c) What is unreasonable about these results?
(d) Which premises are unreasonable or inconsistent? (Note that accurate gravitational measurements can easily detect the effect of nearby mountains and variations in local geology.)
Final Answer
- Mountains are just fuzz on a massive planet. For any single mountain to comprise a significant fraction of the Earth's mass us unreasonable.
- The gravitational force is not 2.00% of the person's weight.
Solution video
OpenStax College Physics, Chapter 6, Problem 42 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Supposedly there's a mountain that's 10 kilometers away from this person and it's exerting a force of gravity on them— the force due to the mountain— of 2 percent of the person's weight. So the person's weight is their mass times gravitational field strength, G, and 2 percent I have written as a fraction as 0.0200. So the force of gravity is the gravitational constant times the mass of the person times the mass of the mountain divided by the distance between them squared and we are told that that equals 0.0200 times mass of the person times g. We can divide both sides by the mass of the person and then divide both sides by g times mass of the mountain and then multiply both sides by r squared and then we end up with mass of the mountain is 0.0200 times g times r squared over G. So that's 0.0200 times 9.80 newtons per kilogram times 10.0 times 10 to the 3 meters squared divided by 6.67 times 10 to the minus 11 newton meters squared per kilogram squared and that gives 2.9 times 10 to the 17 kilograms. Now we are gonna compare this calculated mass for the mountain to the mass of the Earth and a good way to compare them is to divide them. So we have 2.9 times 10 to the 17 kilograms divided by the mass of the Earth which is 5.98 times 10 to the 24 kilograms and we get 4.9 times 10 to the minus 8. Now this is kind of a small number but mountains are just fuzz on an otherwise very massive planet with a huge interior and a crust. And so for any single mountain to have even this fraction of the total mass of the Earth is unreasonable. So the premise that's not correct is more likely the gravitational force is not 2.00% of the person's weight.