Question
Astronomical observations of our Milky Way galaxy indicate that it has a mass of about solar masses. A star orbiting on the galaxy's periphery is about light years from its center. (a) What should the orbital period of that star be? (b) If its period is instead, what is the mass of the galaxy? Such calculations are used to imply the existence of “dark matter” in the universe and have indicated, for example, the existence of very massive black holes at the centers of some galaxies.
Final Answer
a)
b)
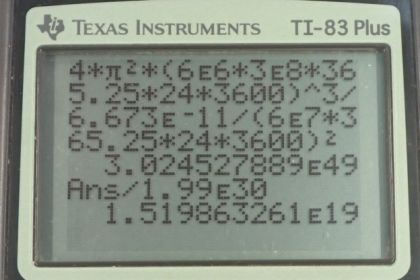
Note: at the end of part (b) there is an incorrect answer due to a button pushing mistake on my calculator where I input 6E6 by mistake, when I should have entered 6E4.Solution video
OpenStax College Physics, Chapter 6, Problem 47 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're going to create an expression to find the orbital period of a star based on the mass of the galaxy that it's orbiting around and the radius of its orbit. So if we return to the beginning of the story, we have that the gravitational force exerted by the center of the galaxy on the star is the centripetal force that causes it to continue in its orbit. Centripetal force is mass times the star's speed squared, divided by its orbital radius and force of gravity is the gravitational constant times the mass of the galaxy, times the mass of the star, divided by its orbital radius squared. So we can simplify this a little bit by dividing both sides by m or multiplying by one over m if you like, and the m's cancel. Then we have g times mass of the galaxy divided by radius squared equals v squared over r. We can substitute for v and say that it's going to be the circumference of its orbit, the size of the circle that it travels around, divided by the time it takes to complete the orbit, its orbital period in other words. Then we'll substitute that in for v. So we have two pi r over T, all squared, and then divided by r. Since I don't like to have fractions within fractions, I'm going to say instead of divide by r, we'll say multiply by one over r. This is four pi squared r squared over T squared when you apply the squared to each of the factors in there and then, what did I do next? I canceled one of the r's here to leave us with four pi squared r to the power of one over T squared. Then we want to solve for T squared. So multiply both sides by T squared and then also multiply by r squared to make the r squared cancel over here and then divide both sides by g m. So, on the left side we have everything canceling except the T squared is going to be left behind. On the right had side we have four pi squared r to the power of three over g m. Then take the square root of both sides to get the orbital period T. So T is going to be the square root of four pi squared r cubed over g m. Then if we want to simplify it a bit more you can take the four pi squared out of the square root sign and call it two pi instead. So two pi times the square root of the orbital radius of the star and it's six times ten to the four light years from the center of the galaxy and we have to convert that into meters, and that's three times ten to the eight meters per second because a light year is the distance the speed of -- some thing travels at the speed of light in a year. So I usually like to abbreviate it as c times y. The units are kind of like -- the letter c by the way is the tip traditionally used for speed of light. So this has units of meters per second because that's what the speed of light is measured in, multiplied by years. So we need to -- we have the meters per second there and then we multiply by one day per year and hour per day and then 3600 seconds per hour in order to get rid of all the time units that are there. So multiplying by 365 and a quarter days per year cancels the years that are here and then -- well, since I said this is c y then I guess if you wanted to be more explicit about things, this would be 3.0 times ten to the eight meters per second per c. Then let's call this c y instead of l y and so now we have three times ten to the eight meters per second for every c and so the c's cancel and then the years canceled from this conversion factor. Then you have 24 hours per day so the days cancel, then times by 3600 seconds per hour and you're left with -- well, these seconds cancel with the seconds that are there and you're left with meters. Good! Then you cube those meters and divide that by the gravitational constant, 6.67 times ten to the minus eleven. Then multiply that by the mass which has to be in kilograms and so we're given an estimate of the mass of the galaxy as eight times ten to the eleven solar masses. So we multiply that by 1.99 times ten to the thirty kilograms for every solar mass. This works out to 8.3 times ten to the fifteen seconds. Now, then part B we're told to well, let's suppose we know what the period is of the star's orbit and we know its orbital radius. Calculate what the mass should be. This mass up here could have been estimated by looking at the number of stars that are in the galaxy and making a calculation or an estimate of the mass based on the amount of stars that you see. But as we'll see in part B, that estimate isn't very good because when you calculate what the mass should be we find that the mass is much larger than what one would assume based on the mass that you see. So the mass we're comparing to is 1.52 times ten to the nineteen solar masses whereas we had 8.0 times ten to the eleven solar masses estimated before. That's many orders of magnitude different and this is the basis for assuming that dark matter must exist because the orbital periods that we observe and the orbital radii require a mass much larger than what one would assume based on the stuff you can see. So there must be stuff there that you can't see. Okay. So, we rearrange this formula to solve for m and so we multiply both sides by m overT squared and end up with this expression here. So that's four pi squared times six times ten to the four light years converted into meters, cubed, divided by the gravitational constant and then this period that we're told to presume which is six times ten to seven years. We have to assume the units are years there -- the text book isn't very clear in this question but we'll assume that they mean years -- then square that after converting to seconds. We end up with 3.02 times ten to the forty-nine kilograms. Then we'll convert that into solar masses in order to compare with the mass based on what's the observable matter in the solar system by multiplying by one solar mass for every 1.99 times ten to the thirty kilograms. This gives 1.52 times ten to the nineteen solar masses. So we can see that the mass that should be there is much larger than the mass we can see.
Comments
Hi, I was wondering why you put 6E6 into the calculator for part b) when it's 6E4 light years? - I got 3.02E43 kg and therefore 1.52E13 solar masses.