OpenStax College Physics, Chapter 19, Problem 22 (Problems & Exercises)

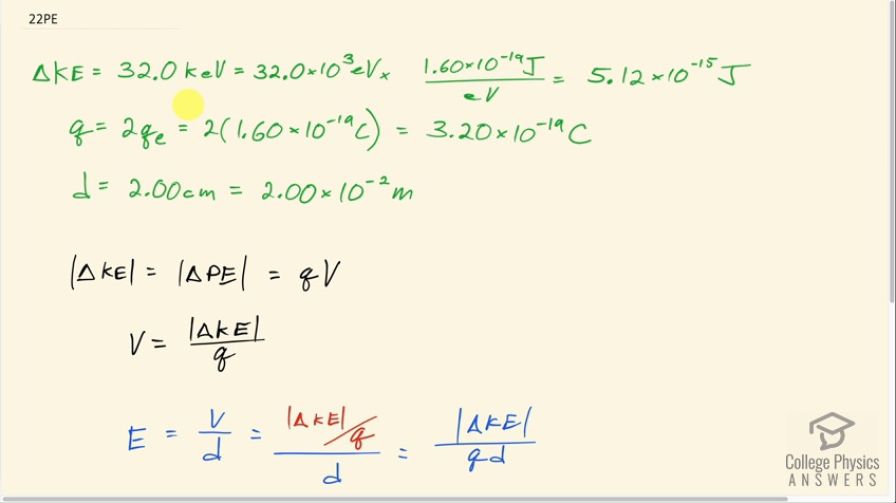
This is College Physics Answers with Shaun Dychko. A doubly-charged ion is accelerated to an energy of 32.0 kiloelectron volts. We are gonna have to do a bunch of things in this preamble here, this section where we write down the things that we know and convert units into mks units—meters, kilograms and seconds. So joules is an mks unit because it contains meters and kilograms and seconds within it and so we are gonna convert these kiloelectron volts into joules. So first we'll write times 10 to the 3 in place of the prefix 'kilo' and we'll take this number of electron volts and multiply it by 1.60 times 10 to the minus 19 joules per electron volt and we end up with 5.12 times 10 to the minus 15 joules. The ion is doubly-charged, which means that its charge is two times the elementary charge so that's 2 times 1.60 times 10 to the minus 19 coulombs which is 3.20 times 10 to the minus 19 coulombs. And the electric field is between plates that are separated by 2.00 centimeters, which is 2.00 times 10 to the minus 2 meters. Okay! So the amount of change in kinetic energy of these ions is the same as the amount of change in their potential energy and I write these absolute value signs here just so that we ignore the negative... I mean, you know... the change in kinetic energy is opposite to the change in potential energy, strictly speaking, so when there's a loss in potential energy that negative change in potential will be multiplied by this negative resulting in a positive amount of change in kinetic energy which is to say that whatever the ion loses in potential energy gains in kinetic energy but I prefer to just keep that idea in mind but not have the negative signs, sort of, get in the way of our work here. So all this is gonna equal the charge times the potential and so this is substituting for the change in potential energy. And this V then, if we divide both sides by q, we can see it's the amount of change in kinetic energy divided by q. Now the electric field is V divided by the separation between the parallel conducting plates and V we just said is the change in kinetic energy per charge and so we can write ΔkE over qd is our electric field. So that's 5.12 times 10 to the minus 15 joules divided by 3.20 times 10 to the minus 19 coulombs times 2.00 times 10 to the minus 2 meters which is 8.00 times 10 to the 5 volts per meter.