Question
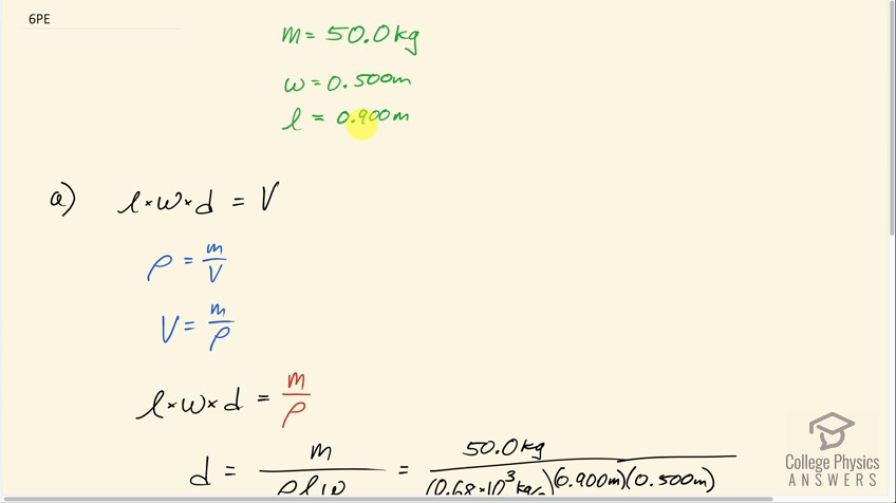
(a) A rectangular gasoline tank can hold 50.0 kg of gasoline when full. What is the depth of the tank if it is 0.500-m wide by 0.900-m long? (b) Discuss whether this gas tank has a reasonable volume for a passenger car.
Final Answer
- . Yes, this is reasonable. This would be a gas tank on a large vehicle.
Solution video
OpenStax College Physics, Chapter 11, Problem 6 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are told that a gas tank is holding 50.0 kilograms of gasoline and it has a width of 0.500 meters and a length of 0.900 meters and it's rectangular shaped so we have these dimensions here— a width and a length— and our question is to find the depth now... something like that. So what is this up and down dimension here that's d, the depth. So the three dimension's multiplied together makes the volume and we don't know what the volume is yet; we know the mass and we also don't know d so we need to figure out what V is and we use the density formula to find it. So density is mass divided by volume and we can rearrange this to solve for V by multiplying both sides by V over ρ and then we have V is mass divided by density. So we can make a substitution for V and replace it with m over ρ instead and then solve this for d by dividing both sides by lw. So we have the depth then is the mass of 50.0 kilograms divided by the density which we can look up in table [11.1] and for 'Petrol'—that's the British word for gasoline— it's 0.68 grams per milliliter... I used the kilograms per cubic meter here. So we can also take these numbers and multiply it by 10 to the 3 kilograms per cubic meter and this gets multiplied by 0.900 meters times 0.500 meters and we end up with 0.16 meters is the depth. So the volume is 74 liters when you divide this mass, converted into grams, by the density of petrol, which is 0.68 grams per milliliter, and then convert the milliliters into liters and that is reasonable for a large vehicle—74 liters.


