Question
There is relatively little empty space between atoms in solids and liquids, so that the average density of an atom is about the same as matter on a macroscopic scale—approximately 103 kg/m310^3 \textrm{ kg/m}^3. The nucleus of an atom has a radius about 10−510^{-5} that of the atom and contains nearly all the mass of the entire atom. (a) What is the approximate density of a nucleus? (b) One remnant of a supernova, called a neutron star, can have the density of a nucleus. What would be the radius of a neutron star with a mass 10 times that of our Sun (the radius of the Sun is 7×108 m7\times 10^{8}\textrm{ m})?
Question by OpenStax is licensed under CC BY 4.0
Final Answer
  1. 1018 kg/m310^{18} \textrm{ kg/m}^3
  2. 2×104 m2\times 10^{4}\textrm{ m}

Solution video

OpenStax College Physics, Chapter 11, Problem 10 (Problems & Exercises)

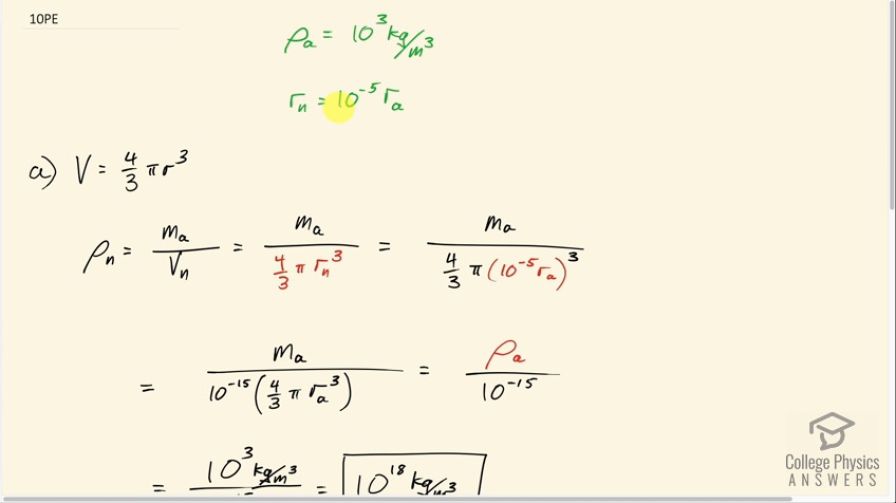
OpenStax College Physics, Chapter 11, Problem 10 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 11, Problem 10 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. In this question, we want to find out what the density of a nucleus is and we are told that the radius of a nucleus is about 10 to the minus 5 multiplied by the radius of the whole atom and that pretty much all of the mass of the atom is in the nucleus and so when we are calculating the density of a nucleus, we can take the mass of the whole atom divided by the volume of the nucleus. And we'll assume that the nucleus is a sphere in which case its volume is four-thirds π times its radius cubed so I make that substitution here. And then replacing r n, which is the radius of the nucleus, with 10 to the minus 5 times the radius of the atom and take this 10 to the minus 5 out of the bracket, cube it and it becomes 10 to the minus 15 and we have this whole thing is the density of an atom and we are told what that density is here. So we have the density of a nucleus is about the density of an atom— 10 to the 3 kilograms per cubic meter— divided by 10 to the minus 15 which is 10 to the 18 kilograms per cubic meter. In part (b), it says what would the radius be of a neutron star assuming it has the same density as a nucleus and it has a mass 10 times that of the Sun? So its mass then is gonna be 10 times 1.99 times 10 to the 30 kilograms so that's 1.99 times 10 to the 31 kilograms and its density is mass divided by its volume— volume being four-thirds πr cubed assuming its a sphere—and we can multiply top and bottom by 3 here... the 3's cancel and we are left with 3M n over 4πr cubed and then we'll solve this for r by multiplying both sides by r cubed over ρ and then take the cube root of both sides. So we have r is a cube root of 3 times mass of the neutron star divided by 4π times its density and its density we are told is the same as that of a nucleus— 10 to the 18 kilograms per cubic meter. So we plug in numbers, raise it to the power of one-third, which is the same as cube rooting, and we get 2 times 10 to the 4 meters would be the neutron star's radius.

Comments

Hi, after you sub in the radius of an atom and take it out of the brackets it was 10^-15*(4/3*_PI_*r(sub_a)^3. I am confused here as to why all of a sudden it is only just 10^-15 and why the r(sub_a) didnt disappear when you subbed in the radius and why after subbing in you did not have to multiply by the 4/3 PI still?? I am probably just missing something silly or not quite understanding a simple concept I am sure but I am going nuts trying to figure out why the answer is the way it is, thanks so much for any help here and I hope your holdidays are going wonderful. You rock man thanks again for the hard work here.

Oh gosh I think I was reading it wrong at first, its making a little more sense keeping the r(sub_a) which is the radius of the of the atom and the radius of the nuculi has a radius 10^-5 that of the radius of the atom.
But I am still confused as to what happeded to all that and why its only 10^-15.