Solution video
OpenStax College Physics, Chapter 23, Problem 8 (Problems & Exercises)
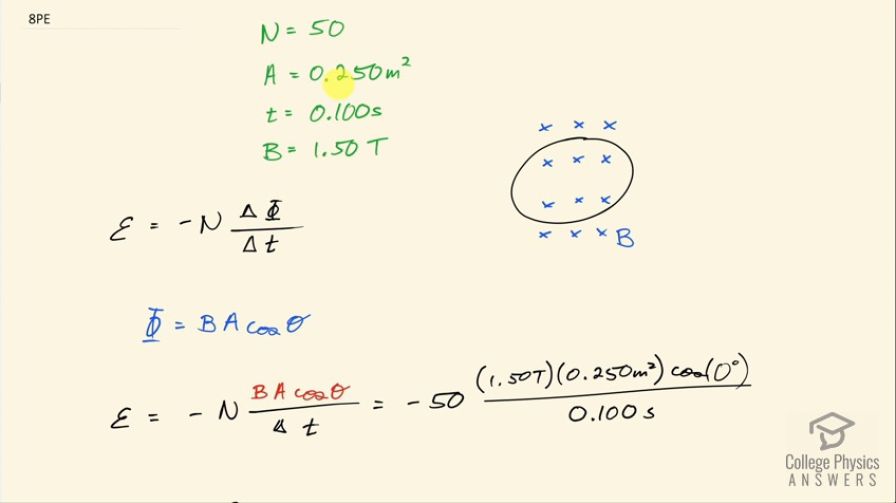
Calculator Screenshots
Comments
I am confused on which aspect would change based on if the direction was out of the page versus into the page. Would the negative sign be taken away since it does not oppose the flux? Thank you.
Thank you for the question amartino,
The negative sign shouldn't be taken too literally here. It's not like in kinematics where the negative sign usually means directed down. In this case, the negative is a reminder that the induced EMF is directed in order to produce an induced magnetic field that opposes the change in the flux through the loop. If the uniform magnetic field was directed out of the page, the induced EMF would be counterclockwise in order to produce an induced magnetic field directed out of the page in order to try replacing the magnetic flux that is decreasing due to the decrease in the area of the loop.
All the best,
Shaun


