Question
Your RL circuit has a characteristic time constant of 20.0 ns, and a resistance of . (a) What is the inductance
of the circuit? (b) What resistance would give you a 1.00 ns time constant, perhaps needed for quick response in an oscilloscope?
Final Answer
Solution video
OpenStax College Physics, Chapter 23, Problem 70 (Problems & Exercises)
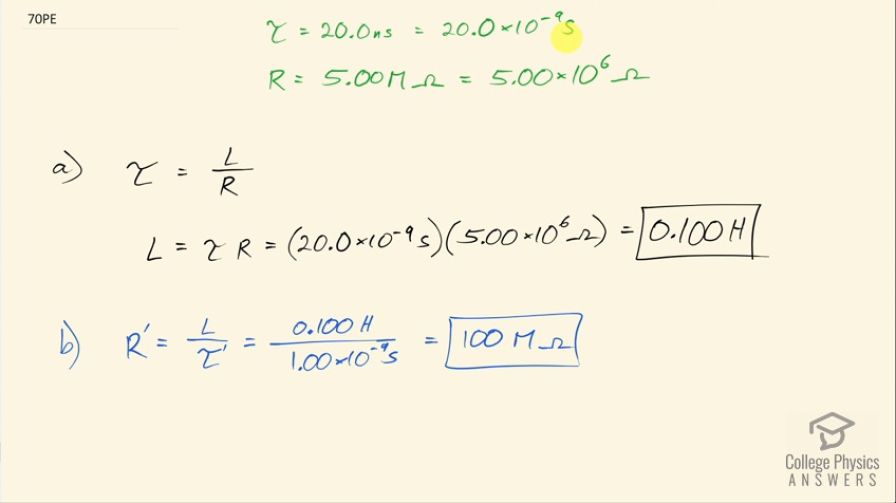
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The characteristic time constant of an LR-circuit is 20.0 nanoseconds, which is 20.0 times 10 to the minus 9 seconds and it has a resistance of 5.00 megaohms, which is 5.00 times 10 to the 6 ohms. The question in part (a) is asking what is the inductance of the circuit? So we know that the characteristic time constant is the inductance divided by the resistance so we can solve this for L by multiplying both sides by R so L then is τ times R. So that's 20.0 times 10 to the 9 seconds times 5.00 times 10 to the 6 ohms which is 0.100 henries. Part (b) says what should the resistance be in order to have a time constant of 1.00 nanosecond? So we rearrange this formula to solve for R but I am calling it R prime because it's a new resistance, it's not the same R as we had in part (a) and likewise, τ gets labeled with this prime on it to indicate that it's a different time constant than we were dealing with before but L is the same, it's the same circuit otherwise with the same inductance. So R prime then is the inductance that we calculated in part (a) divided by this new time constant that we desire. So that's 0.100 henries divided by 1.00 nanosecond written as 1.00 times 10 to the minus 9 seconds and that's 100 megaohms woud be needed to create this time constant given this inductance.
