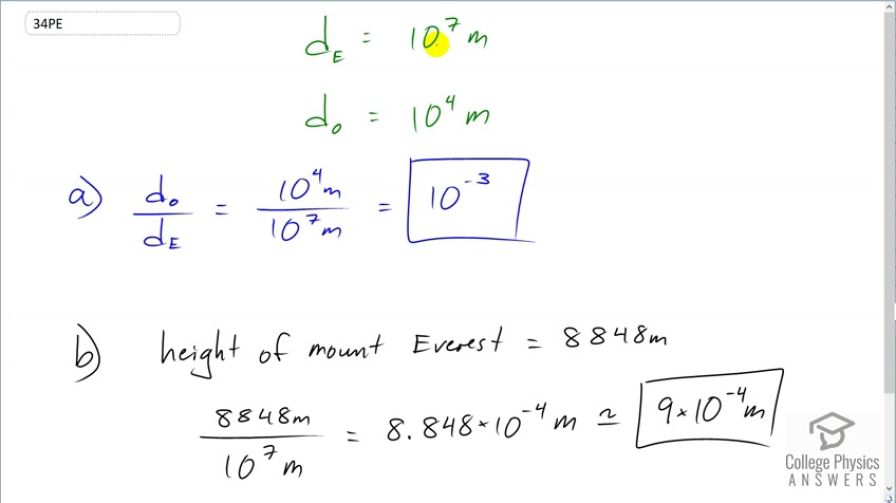
Question
(a) What fraction of Earth's diameter is the greatest ocean depth? (b) The greatest mountain height?
Final Answer
Solution video
OpenStax College Physics, Chapter 1, Problem 34 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. What fraction of the Earth's diameter is the greatest ocean depth? We need to look up these numbers in our data table in chapter 1 and the diameter of Earth is 10 to the 7 meters and the deepest ocean is 10 to the 4 meters so we have 10 to 4 divided by 10 to the 7 giving us 10 to the minus 3. In part (b), what fraction is the height of Mount Everest of the diameter of the Earth? Well, it says the highest mountain and the highest mountain is Mount Everest which is 8848 meters. So we take that height, divide it by 10 to the 7 and we get 8.848 times 10 to the minus 4; there's no units in there by the way, oops, no units at all because we are dividing meters by meters. So the question is how many significant figures should this have; we have lots of significant figures in that measurement of the Mount Everest height but in this denominator, there's only one significant figure because when you see 10 to the 7, you can think of it as 1 times 10 to the 7 and this has one significant figure and so we should have one significant figure in our answer then so we'll call this 9 times 10 to the minus 4 is the ratio of the highest mountain to the Earth's diameter.
