Question
A young woman with normal distant vision has a 10.0% ability to accommodate (that is, increase) the power of her eyes. What is the closest object she can see clearly?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 26, Problem 14 (Problems & Exercises)
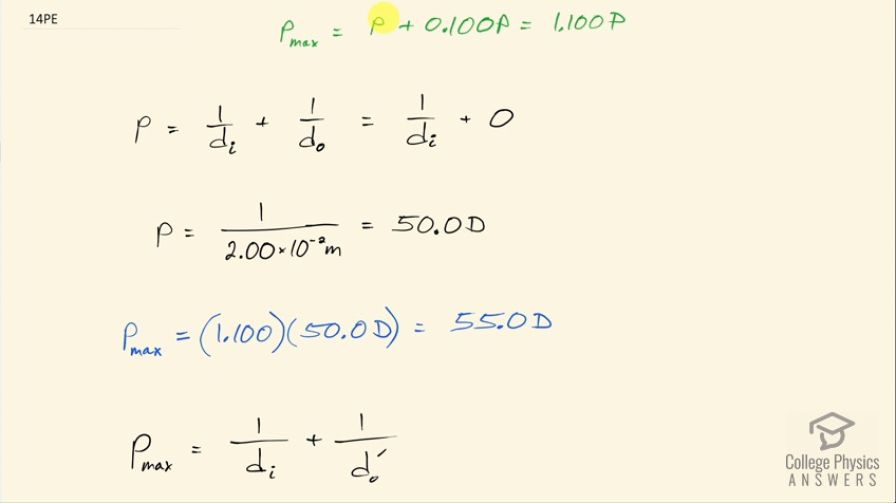
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A woman with normal distant vision is able to accommodate by 10 percent and the question is what is the nearest object that they can see so to figure that out, we need to know what is the maximum possible power that their eye can have? And they have normal distant vision so we can take that normal distant vision and we'll figure out that that's 50.0 diopters in a second and that normal distant power can be increased by 10.0 percent so we add 0.100 multiplied by the power to it and it works out to 1.100 times the distant power. So let's figure out what this power at a distance is for normal vision and so that's 1 over image distance plus 1 over object distance— that's power in general— and in this case, the object distance is infinity and so this fraction becomes zero. So the far-point for normal vision is infinity and this is 1 over image distance, which is the distance between the lens and the retina for the regular person, which is 2.00 centimeters and so the power for normal distant vision then is 1 over 2.00 times 10 to the minus 2 meters, which is 50.0 diopters. And so the maximum power then when the person's eye is accommodating or increasing its power is 1.100 times that normal 50.0 diopters for distant vision and this is 55.0 diopters. So what's the object distance then when the power of the eye is 55.0 diopters? So this maximum power is 1 over the same image distance as it was before because the lens-retina distance doesn't change so there's no prime here intentionally whereas this object distance here is a new object distance—it's not the same d o as before— this is the object distance when looking at something at their near-point and so it gets a prime there to distinguish it from this d o. Now we are solving for d o prime; first, we'll subtract 1 over image distance from both sides and so 1 over the object distance at its near-point, prime in other words, equals the maximum power minus 1 over d i and then we raise both sides to the negative 1 to solve for d o prime. So the near-point then is the maximum power minus 1 over image distance all to the negative 1, it's 55.0 diopters minus 1 over 2.00 times 10 to the minus 2 meters— lens-retina distance— all to the negative 1, which is 0.200 meters which is 20.0 centimeters. So this person can see something at its closest at 20.0 centimeters away which is better than normal, which is 25.0 centimeters.
