OpenStax College Physics, Chapter 33, Problem 3 (Problems & Exercises)

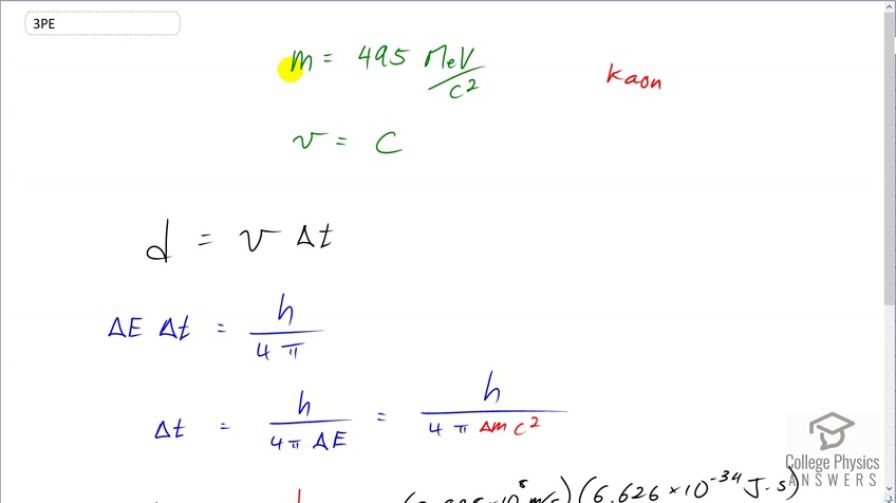
This is College Physics Answers with Shaun Dychko. The K meson or also known as a kaon is another mediator of the strong nuclear force and they have a mass of 495 megaelectron volts per c squared and the question is over what distance can this virtual particle mediate the force; in other words, over what distance does this force apply? And we know the answer is going to be very small on the order of the size of a nucleus or nucleons because it's the strong nuclear force that applies only inside the nucleus. We'll give it the maximum possible speed that one can imagine for this virtual particle so that we can maximize its possible distance over which it can act; we'll take this speed to be c and of course, nothing can actually reach this speed but this is a virtual particle and it can break the laws for a duration that is less than the smallest theoretical uncertainty in any measurement of it. So we'll turn to the Heisenberg uncertainty principle in order to figure out what that uncertainty is. So the distance over which it acts is going to be its speed multiplied by its lifetime and its lifetime at the most will be the minimum uncertainty in any measurement of it. So Heisenberg uncertainty principle says that the uncertainty in its energy multiplied by uncertainty in its lifetime is going to be Planck's constant over 4π. And so we'll divide both sides by uncertainty in the energy and we solve for Δt. And we take this uncertainty in energy to be the mass energy of the particle. So we substitute all of this in for Δt in our formula for the distance over which the force will act. And so we have v times h over 4π times Δm c squared. So we have the speed of light times Planck's constant divided by 4π times the mass of this particle converted into well we multiply it by c squared. So we are given 495 megaelectron volts per c squared is the mass and then we multiply that by c squared and we are left with electron volts which we then convert into joules because we want to have mks units in this formula and we end up with 0.2 femtometers is the distance that this virtual particle can travel. Yeah and so that's an expected order of magnitude the size of nucleons.