Question
(a) What is the uncertainty in the energy released in the decay of a due to its short lifetime?
(b) Is the uncertainty in this energy greater than or less than the uncertainty in the mass of the tau neutrino? Discuss the source of the uncertainty.
Final Answer
- u
- The uncertainty in energy is much less than the uncertainty in the mass of the tau neutrino.
Solution video
OpenStax College Physics, Chapter 33, Problem 20 (Problems & Exercises)
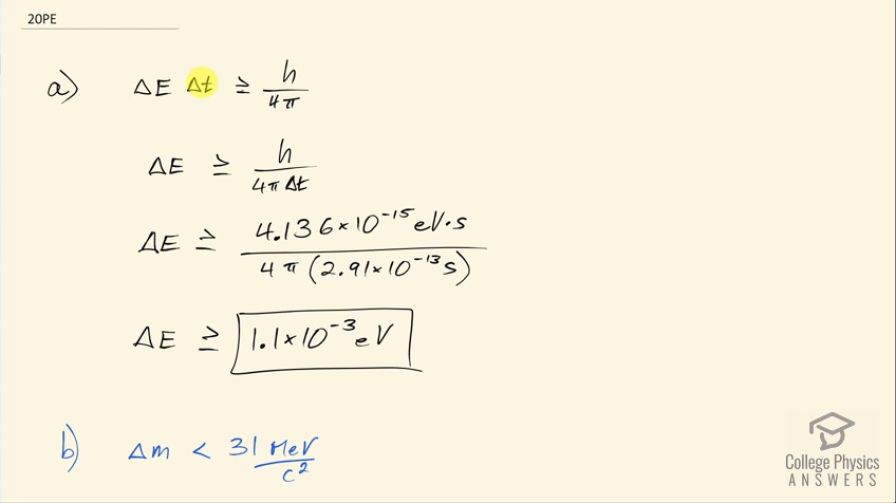
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to calculate the uncertainty in the energy released in the decay of a tau particle. We know that uncertainty in energy multiplied by uncertainty in time is greater than or equal to Planck's constant over 4π— this is the Heisenberg uncertainty principle— and we can solve for ΔE by dividing both sides by Δt. So the uncertainty in energy then is at least Planck's constant over 4π times uncertainty in time. Now if we take the maximum possible uncertainty in time then that will minimize or give us the smallest amount of uncertainty in energy so by maximizing the denominator, we minimize the quotient in other words and the maximum possible uncertainty in time we can have is the lifetime of the particle and the lifetime of the tau particle is 2.91 times 10 to the minus 13 seconds so we substitute that in for Δt here. Planck's constant we take to have units of electron-volt seconds and this all works out to the uncertainty in energy being greater than or equal to 1.1 times 10 to the minus 3 electron volts. Part (b) asks if the uncertainty in the energy is greater than or less than the uncertainty in the mass of the tau-neutrino? Now the tau-neutrino has a mass maybe of 0 but that's not quite certain this number here—31— reflects the uncertainty in the mass of the tau-neutrino that's 31 megaelectron volts per c squared. And so if we write this number as a decimal instead of scientific notation, we can more easily compare it to this number, which we also write well, in electron volts per c squared so we have the same units comparing to each other. So we have 0.0011 electron volts per c squared is the uncertainty in the energy and that's much much less than the mass of 31 times 10 to the 6 electron volts per c squared. So the uncertainty in the energy is much much less than the rest energy of the tau-neutrino.
