Question
In supernovas, neutrinos are produced in huge amounts. They were detected from the 1987A supernova in the Magellanic Cloud, which is about 120,000 light years away from the Earth (relatively close to our Milky Way galaxy). If neutrinos have a mass, they cannot travel at the speed of light, but if their mass is small, they can get close. (a) Suppose a neutrino with a mass has a kinetic energy of 700 keV. Find the relativistic quantity for it. (b) If the neutrino leaves the 1987A supernova at the same time as a photon and both travel to Earth, how much sooner does the photon arrive? This is not a large time difference, given that it is impossible to know which neutrino left with which photon and the poor efficiency of the neutrino detectors. Thus, the fact that neutrinos were observed within hours of the brightening of the supernova only places an upper limit on the neutrino's mass. (Hint: You may need to use a series expansion to find v for the neutrino, since its is so large.)
Final Answer
- . Instead of an approximation, consider using a spreadsheet such as this one since spreadsheets can handle numbers with much greater precision.
Solution video
OpenStax College Physics, Chapter 33, Problem 50 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
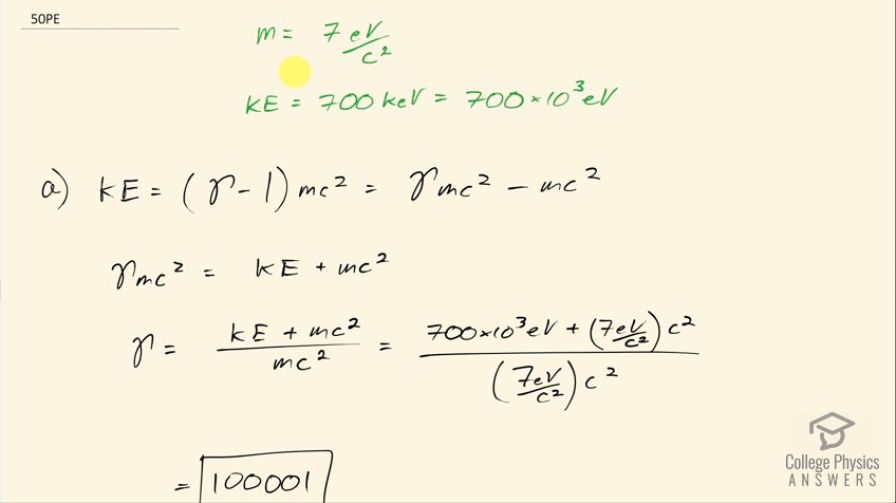
This is College Physics Answers with Shaun Dychko. A Supernova occurred in the Magellanic Cloud in 1987 and that cloud is 120,000 light years away and some neutrinos were detected on Earth as well as light photons, you know, light from the Supernova was also detected but there's a time difference between when they were detected. The neutrinos were slightly slower and that's on account of their mass; to the extent that they have mass, they are not capable of traveling at the speed of light. So we are going to estimate based on kinetic energy of 700 kiloelectron volts for the neutrinos and an estimated mass of 7 electron volts per c squared, what is the time difference between receiving the photons versus receiving the neutrinos? The question in part (a) asks what is this Lorentz factor γ? So we know that kinetic energy is γ minus 1 times rest mass times c squared and then we can expand the bracket by multiplying through by mc squared here and we have γmc squared minus mc squared, we'll add mc squared to both sides and we have γ times mc squared equals kinetic energy plus mc squared then divide both sides by mc squared and then we get γ is kinetic energy plus mc squared all over mc squared and that's 700 times 10 to the 3 electron volts plus 7 electron volts per c squared times c squared divided by 7 electron volts per c squared times c squared and this works out to 100001— that's the value of gamma. In part (b), we have to find this time difference. Well, let's first figure out what is the distance and this is a distance of 120000 light years, which means it's the distance that light would travel in 1 year times 120000. So this l represents the speed of light and this y represents years so sometimes, I like to abbreviate this as c.y— the speed of light multiplied by a time period of 1 year. So we are going to turn this into units of c times s and this is also a bit strange because, you know, normally, I would convert this into a distance of meters but I am not going to do that here because I want this speed of light... I want this distance as a factor of or a factor times the speed of light because the speed of light was going to cancel conveniently later on. So we are just going to convert the year unit though into seconds so we multiply by 365.25 days per year and then 24 hours per day and then 3600 seconds per hour and we are left with 3.786912 times 10 to the 12 times the speed of light-seconds. Alright! So if that seems strange well, it's just as strange as light years and this is now just light seconds. Okay! The time for the neutrino to travel is going to be this distance divided by the speed of the neutrino and we need to solve for v in this expression for the Lorentz factor gamma. So we have... we can square both sides and so we have γ squared is 1 over 1 minus v squared over c squared then multiply both sides by 1 minus v squared over c squared divided by γ squared and then we get 1 minus v squared over c squared equals 1 over γ squared and then we'll add v squared over c squared to both sides and subtract 1 over γ squared from both sides then switch the sides around and then we get v squared over c squared equals 1 minus 1 over γ squared, multiply both sides by c squared and you get this line, take the square root of both sides and you have v then is the speed of light times square root 1 minus 1 over γ squared. And I am not going to substitute in a number for c, I am just going to leave it as the factor c and for the same reason that I left this factor c in this distance as well, it's going to cancel away later on. So we have the square root of 1 minus 1 over 100001 squared is this number and this number comes from spreadsheet program Excel because the calculator isn't capable of handling so many digits but but Excel spreadsheet can so this is square root 1 minus 1 over 100001 squared and that makes 0.9 with ten 9s a 5 and then 0001 so it's that factor times c is the speed. Okay! Now the time difference then is going to be the time it takes for the neutrino to arrive minus the time it takes for a photon to arrive traveling at the speed of light c. So that's the distance divided by the speed of the neutrino minus the distance divided by the speed of light and we can factor the d out and we have d times 1 over speed of the neutrino minus 1 over speed of light. Now I don't want to write in the number for the speed of light because we are dealing with such small... we are dealing with numbers that are so precise I would be introducing a problem by entering in a speed for the speed of light unless I had you know, this many digits in the speed of light which I don't feel like writing down because that's just inconvenient to write down so many digits and I did it here but I don't want to do it for the speed of light as well and there's a way to get around that issue by multiplying this by the number 1 but number 1 is going to look funny— it's going to look like c over c and then leave the c in the denominator for this fraction and then multiply the top c into the brackets. So we have d over c times c over the speed of the neutrino c over c, which is 1. And this is convenient to have this expression because we have c divided by some factor multiplied by c and so the c's cancel and then this distance is some factor multiplied by c which cancels with this c here and we are left with seconds in our units here times 1 over this big number well, it's a small number but, you know, many digits... anyhow... minus 1. Now this again is something that a calculator brain can't really handle so we turn to the spreadsheet and it's going to be this number here, this time it was a distance but then the c canceled with the c in the denominator here so it's 3.786912 times 10 to the 12 seconds— that's this part here— times 1 over this denominator here (whoops) which is the cell A1 minus 1. So I have plugged this into the Excel and it gives the answer 189 so that's 189 seconds. So there's 189 seconds between when the photons are detected from the Supernova and the neutrinos are then detected 189 seconds later.