OpenStax College Physics, Chapter 33, Problem 46 (Problems & Exercises)

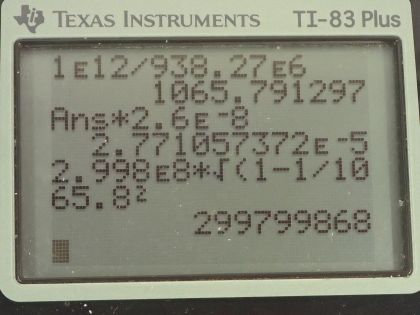
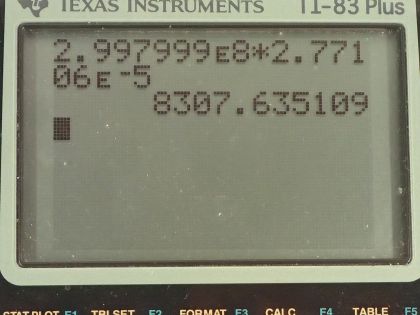
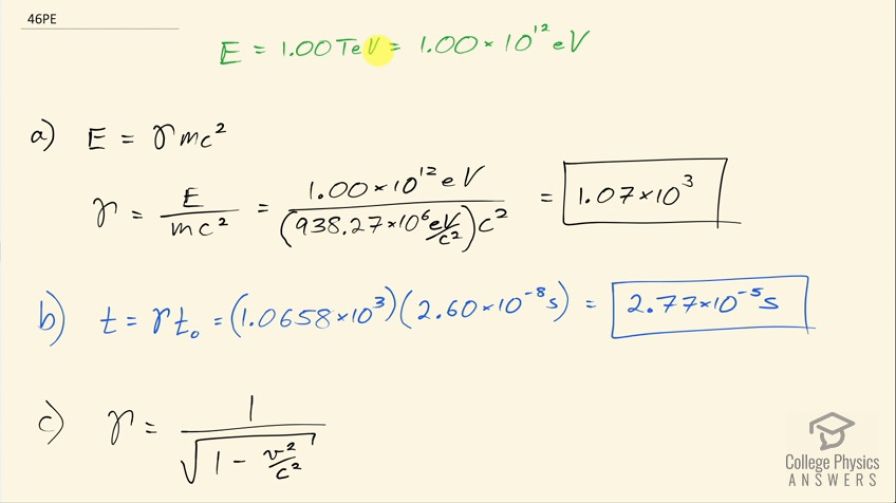
This is College Physics Answers with Shaun Dychko. We suppose that protons at the Fermilab are accelerated to an energy of 1.00 teraelectron volt, which is 1.00 times 10 to the 12 electron volts what will be the Lorentz factor in that case, this γ? And we know that the total energy of a particle equals γ times its rest mass times c squared so we can divide both sides by mc squared to solve for γ. So γ then is the total energy divided by mc squared. So that's 1.00 times 10 to the 12 electron volts divided by 938.27 megaelectron volts per c squared— this is the mass of a proton because we are told that these are protons that are being accelerated and I have written it in units of electron volts here in order to match with the electron volts in the numerator— and then we multiply this by c squared, we get 1.07 times 10 to the 3 is the Lorentz factor γ. Part (b) asks if such a proton created a pion—a pi plus particle— having the same speed, how long would its life be in the laboratory? So by saying it has the same speed that means it has the same value for γ because gamma is 1 over square root 1 minus v squared minus c squared so γ represents the speed and so the relativistic lifetime of this particle will be this Lorentz factor γ multiplied by the lifetime that the particle has in its own reference frame or when it's at rest it will have this proper time, this proper lifetime t naught and we need to look up in table [33.2] what that t naught is. So for a pion, it's 2.60 times 10 to the minus 8 seconds is its lifetime. So we multiply that lifetime by the value for gamma that we calculated in part (a) and we get 2.77 times 10 to the minus 5 seconds Part (c) asks how far could this particle travel? So we should figure out what its speed is based on knowing what γ is. So γ is 1 over square root 1 minus v squared over c squared, first we will square both sides and so we get γ squared equals 1 over 1 minus v squared over c squared and then multiply both sides by 1 minus v squared over c squared and then we get this line here also dividing both sides by γ squared. So we have 1 minus v squared over c squared equals 1 over γ squared and then add v squared over c squared to both sides and subtract 1 over γ squared from both sides then switch the sides around and we have v squared over c squared equals 1 minus 1 over γ squared then multiply both sides by c squared and you get this line here then take the square root of both sides and we get the speed then is c times the square root of 1 minus 1 over γ squared. So that's 2.998 times 10 to the 8 meters per second times square root 1 minus 1 over 1065.8 squared and this works out to 2.997999 times 10 to the 8 meters per second. So we take that speed, multiply it by its lifetime in the laboratory reference frame and that works out to 8.31 kilometers.