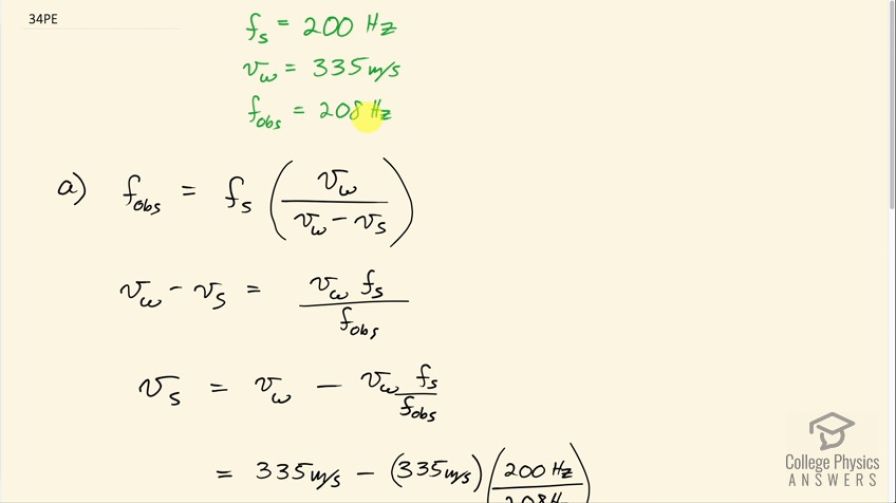
Question
A commuter train blows its 200-Hz horn as it approaches a crossing. The speed of sound is 335 m/s. (a) An observer waiting at the crossing receives a frequency of 208 Hz. What is the speed of the train? (b) What frequency does the observer receive as the train moves away?
Final Answer
Note: At 1:06 I mis-spoke by saying "we are left with ", whereas I should have said "we are left with ". What's written is correct.
Solution video
OpenStax College Physics, Chapter 17, Problem 34 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Prev
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A train is approaching an observer and the observer notices a frequency of 208 hertz from the train's whistle. The train is known to emit a frequency of 200 hertz so that's the frequency of the source and the speed of the sound waves is 335 meters per second. So the question with what speed is the train moving? So the frequency observed equals the frequency emitted by the source multiplied by the speed of sound divided by the speed of sound minus the speed of the source and the source is the train in this case so our job is to solve for v s. So we are gonna multiply both sides by v w minus v s and divide both sides by f observed and we do that on both sides. Then on the left side, we are just left with this numerator v w minus v s and the f obs cancels there and on the right hand side, this binomial is what cancels. So we are left with v wf s divided by f obs and then we add v s to both sides and then subtract v wf s over f obs and then switch the sides around as well and we have the speed of the source, which is the train, equals the speed of sound minus the speed of sound times the frequency of the source divided by frequency observed. So that's 335 meters per second minus 335 times 200 hertz— emitted by the train— divided by 208 hertz—observed by the person that the train is approaching— and that equals 12.9 meters per second is the speed of the train. Now part (b) we are asked what frequency will be observed after the train is finished passing and is now going away from the observer. So in that case, we have the frequency observed is the frequency emitted by the source times the speed of sound divided by speed of sound plus the speed of the source. And we know that we need a plus sign here because from experience, we know that the sound of the train will be lower after its passing or after it's going away from us and so the way to get a lower frequency is to have this denominator bigger than the numerator that will make this fraction less than 1 which will reduce this source frequency by multiplying it by something less than 1 so the frequency observed will be lower as we expect. So we have 200 hertz times 335 meters per second divided by 335 plus the speed that we found in part (a) with lots of decimals so 12.8846 meters per second... this works out to 193 hertz is what would be observed when the train is passing or passed and going away from the observer.