Question
A crude approximation of voice production is to consider the breathing passages and mouth to be a resonating tube closed at one end. (See Figure 17.29.) (a) What is the fundamental frequency if the tube is 0.240-m long, by taking air temperature to be ? (b) What would this frequency become if the person replaced the air with helium? Assume the same temperature dependence for helium as for air.
Final Answer
Solution video
OpenStax College Physics, Chapter 17, Problem 52 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
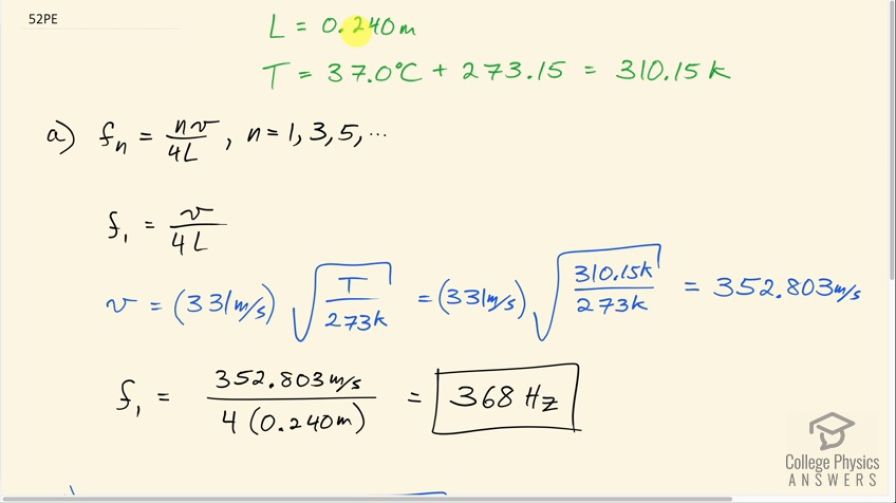
This is College Physics Answers with Shaun Dychko. We are going to assume that a voice is produced by a resonating tube that's closed at one end with a length of 0.240 meters and of course being inside your body, the temperature of the air in your throat will be 37.0 degrees Celsius which we convert into Kelvin by adding 273.15. Part (a) is asking what would the fundamental frequency be and we have this formula for the nth frequency, the nth harmonic is n, where n is a odd natural number— 1, 3, 5 and so on— multiplied by the speed of sound divided by 4 times the length of the tube—this is assuming the tube is closed at one end. So the fundamental frequency will be with n replaced by the number 1 and so it's going to be v over 4L. Now in order to calculate this frequency, we'll need to know what the speed of sound is. So let's multiply 331 meters per second by the square root of the absolute temperature divided by 273 Kelvin. So that's 331 meters per second times square root of 310.15 Kelvin divided by 273 which is 352.803 meters per second. So we take that speed, divide by 4 times the length of the throat of 0.240 meters and that works out to 368 hertz. Now if the throat was full of helium, we would expect a frequency that's higher based on experience of maybe doing this or hearing somebody inhale a bit of helium before speaking their voice will go higher pitch so we expect the fundamental to be a bigger number than 368 hertz. We need to know what is the speed of sound in the case of helium and we'll call that v prime and we are told that to assume that it is the same temperature dependence for helium as there is for regular air. So we look up in table [17.1] what the speed of sound is in helium at 0 degrees Celsius and that's 965. So that's the number we use instead of 331 that we used before with air in calculating the speed of the sound. So that's 965 meters per second multiplied by square root of 310.15 Kelvin over 273 and that's 1028.565 meters per second. So we take that new speed, divide it by 4 times the same throat length of 0.240 meters gives a new fundamental frequency of 1.07 kilohertz.

