Question
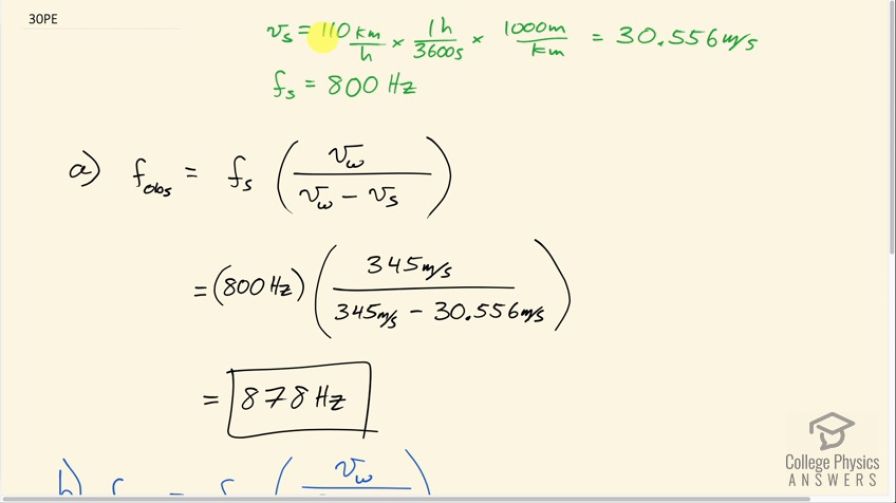
(a) What frequency is received by a person watching an oncoming ambulance moving at 110 km/h and emitting a steady 800-Hz sound from its siren? The speed of sound on this day is 345 m/s. (b) What frequency does she receive after the ambulance has passed?
Final Answer
Solution video
OpenStax College Physics, Chapter 17, Problem 30 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The ambulance is the source of the sound and so its velocity gets labeled v s for sound; it's going at 110 kilometers an hour and we have to convert that into meters per second by multiplying by 1 hour for every 3600 seconds times 1000 meters for every kilometer... this works out to 30.556 meters per second. The frequency of the source is 800 hertz; now when the ambulance is approaching the stationary observer, the frequency of the observer we'll notice is the source frequency times the speed of the sound wave divided by the speed of the sound minus the speed of the source. So that's 800 hertz times 345 meters per second divided by 345 minus 30.556 meters per second and this is 878 hertz and this gives an answer greater than the source frequency as we expect with the ambulance approaching the observer and causing more wavelength to pass the observer per second as a result of the ambulance moving towards it while emitting the sound. Alright! Now after the ambulance is going away from the person, we have a plus sign in the denominator so we have 800 hertz times 345 meters per second divided by 345 plus 30.556 meters per second this gives a frequency of 735 hertz noticed by the observer when the ambulance is moving away from the observer after passing.

