OpenStax College Physics, Chapter 18, Problem 55 (Problems & Exercises)

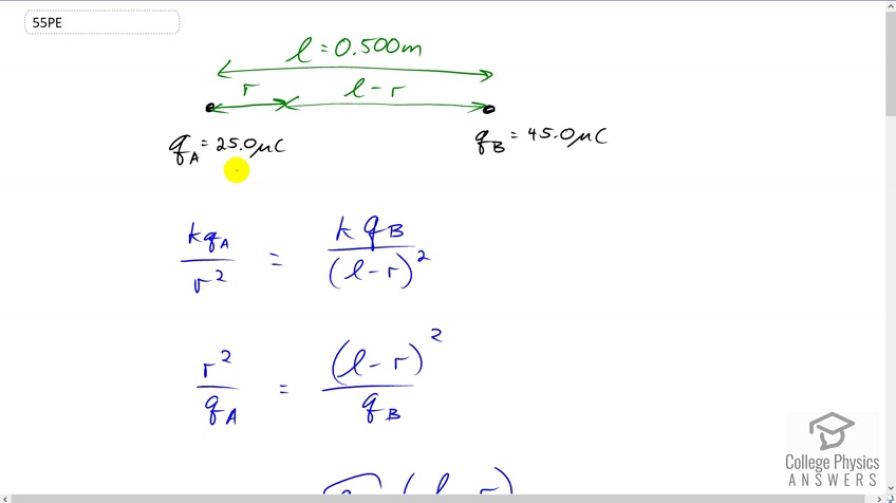
This is College Physics Answers with Shaun Dychko. We're going to find the position between these two charges such that the electric field is zero. So charge a on the left is 25 micro Coulombs and charge b is 45 micro Coulombs. And our position is going to be here somewhat to the left of center so that we're closer to this smaller charge and because the charge is smaller, we need to be closer to it in order to get a greater electric field from it versus this charge b, which has a greater magnitude and we're a bit farther away from it. So these two charges are separated by distance l which is 0.5 meters and for this position here, we'll say it's distance r from qa. And we'll express the distance from qb in terms of l and r. And so we'll write this distance as l for full separation minus r, the distance from qa. So the electric field due to charge a is Coulombs Constant times qa divided by r squared. And then, that's going to equal the electric field due to charge b which is going to be k times qb over l minus r squared. And we'll cancel the Coulombs Constant of both sides and then take the reciprocal of both sides which is the same as raising it to the exponent negative one and we get our unknown at the top. So we have r squared over qa equals l minus r squared over qb. Then, we'll multiply both sides by qa and then take the square root of both sides and we get r equals square root qa over qb times l minus r to the power of one. And then, we'll distribute the square root into the brackets there and we'll multiply each term inside by the factor here. So we have r equals l times square root qa over qb minus r times square root qa over qb. And then collect the r terms on the left side by adding r times square root qa over qb to both sides. And we get r plus r times square root qa over qb equals l times square root qa over qb. Then we factor out the common factor r, and we get this line here. And then divide both sides by this bracket. And then divide the right hand side by that, too. Then, we get the distance r is l times square root qa over qb divided by one plus square root qa over qb. So that's 0.5 meters separation between the charges times the square root of charge a which is 25 micro Coulombs divided by charge b which is 45 micro Coulombs. And normally I would change these units into base units and write times ten to the minus six Coulombs but in this particular case that isn't necessary because we're dividing here and when you divide units that are the same, they cancel. And so the only important thing is that they are the same units. And so you could've gone times ten to the minus six Coulombs on for each of this units and that will be fine, but it's just unnecessary writing. And so I didn't do that. And then we divide by one plus square root 25 micro Coulombs over 45 and that gives 0.214 meters which is from the 25 micro Coulomb charge between the charges. So, this position here which is 0.241 meters from charge a, this is where the electric field will be zero. So it's about approximately one quarter of the way between the two charges. It's almost half way, actually. Part b says, what would the electric field be exactly in the middle. Well, the net electric field will be that due to charge a plus electric field due to charge b and the sign here will indicate the direction. And so we're going to take positive to the right and so the field due to charge a is going to be positive and the field due to charge b will be negative since charge b will be creating a field to the left. And so we have kqa over the distance which is the separation divided by two squared minus kqb over l over two squared. Well the denominator here, it becomes l squared over four and then we can multiply top and bottom by four and then this four here is cancel and this one and we're left with four in the numerator. And that's a common factor between the two terms along with k and l squared in the denominator which we can factor out. So we have four k over l squared times the difference between the charges qa minus qb. So we're substitute in numbers now four times Coulombs Constant 8.988 times ten to the nine newtons meter squared per Coulomb squared divided by 0.5 meters squared times the difference between the charges. And I did change the units into times ten to the minus six now because we're mixing Coulombs here with the Coulombs there. And so we have to be consistent with our units. So 25 times ten to the minus six Coulombs minus 45 times ten to the minus six Coulombs. This works out to negative 2.88 times ten to the six newtons per Coulomb. This negative sign indicates the direction to the left. And so electric field is 2.88 times ten to the six newtons per Coulomb away from the 45 micro Coulomb charge.