Question
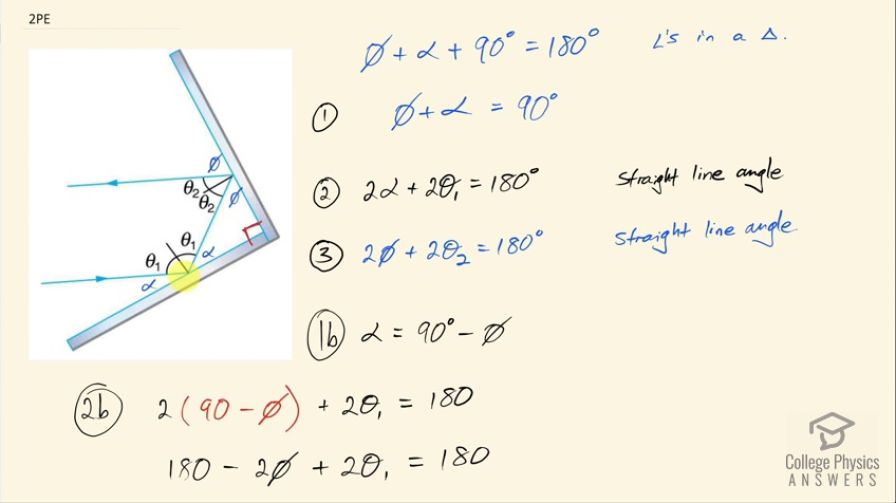
Show that when light reflects from two mirrors that meet each other at a right angle, the outgoing ray is parallel to the incoming ray, as illustrated in the following figure.
Final Answer
Please see the solution video.
Solution video
OpenStax College Physics, Chapter 25, Problem 2 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. These two mirrors we are told are at right angles to each other and there's an incident ray that hits this mirror and the ray bounces off and then hits the other mirror and is reflected back in a direction that is parallel to the incident ray and we have to show that this reflected ray here is parallel to this incident ray down here. so we want to be able to go like this, and put these little parallel signs on there and we are going to do that by showing that these interior angles on the same side of a transverse that intersects these two lines... these interior angles add up to 180 degrees; if we can show that that's true then it must mean that these two lines are parallel. Okay! So... first we'll show that... we'll say that this angle Φ plus this angle α have to add up to 90 and that's true because they are all part of this right triangle here and the total angle in a right triangle is 180 degrees; one of the angles is 90 so we take 90 away from both sides and we are left with 90 degrees for the sum of Φ and α. And point (2) here is that 2 times α plus 2 times Θ 1 equals 180 degrees and that's true because these total the straight line angle which is always 180 degrees. So I am writing the reasons in the right hand column here. Point (3) says that 2 times Φ plus 2 times Θ 2 is also 180 degrees also because of a straight line angle. So now we are going to work with all three of these to show that 2 times Θ 1 plus 2 times Θ 2 is 180. So equation 1 version b says that α is 90 minus Φ because we can subtract Φ from both sides here and then we can substitute that into equation (2) replacing α with this instead and we do that in red here so this is equation 2 version b. So we have 2 times 90 minus Φ plus 2 times Θ 1 equals 180 so that's 180 minus 2Φ plus 2Θ 1 equals 180 and from this we can say that 2Φ equals 2Θ 1 because you can add 2Φ to both sides and then subtract 180 from both sides and we are left with this here and this we can use to replace the 2Φ in equation (3). So 2Φ is 2Θ 1 so we can replace 2Φ with 2Θ 1 here and now we have shown that 2Θ 1 plus 2Θ 2 has to add up to 180 degrees and that means that these lines are parallel because when you have a transverse between two lines and the interior angles on the same side of that transverse add to 180 degrees, it must be so that the lines this transverse is intersecting are parallel.