Question

(a) When rebuilding her car's engine, a physics major must exert 300 N of force to insert a dry steel piston into a steel cylinder. What is the magnitude of the normal force between the piston and cylinder? (b) What is the magnitude of the force would she have to exert if the steel parts were oiled?

Question by OpenStax is licensed under CC BY 4.0

Final Answer

$1000 \textrm{ N}$
$30 \textrm{ N}$

Solution video

OpenStax College Physics, Chapter 5, Problem 2 (Problems & Exercises)

Name: OpenStax College Physics Solution, Chapter 5, Problem 2 (Problems and Exercises)
Uploaded: 2019-11-15T13:48:51-08:00
Duration: 110 s
Description: (a) When rebuilding her car's engine, a physics major must exert 300 N of force to insert a dry steel piston into a steel cylinder. What is the magnitude of the normal force between the piston and cylinder? (b) What is the magnitude of the force would she have to exert if the steel parts were oiled?

In order to watch this solution you need to have a subscription.

Start free trial Log in

vote with a rating of votes with an average rating of .

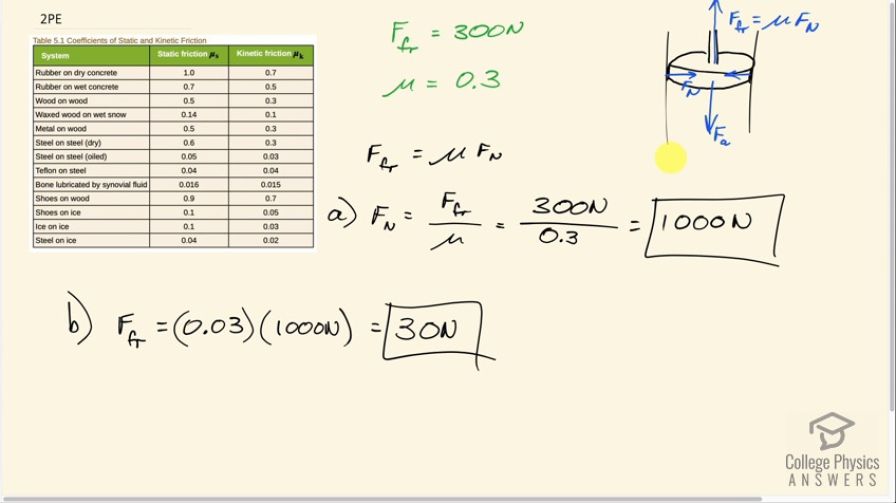
Calculator Screenshots

Video Transcript

This is College Physics Answers with Shaun Dychko. A Physics major is pushing a piston into a cylinder and we are told the piston and cylinder are both made out of steel and they are dry and so those are pieces of information we need when we look at table [5.1] and we look at steel on steel—when it's dry— we have a coefficient of static and kinetic friction. In this case, the piston is sliding within the cylinder and so it's kinetic friction that we want to use and we are going to figure out what the normal force is being applied in total between the surface of the cylinder and the edge of the piston. Because the friction force that's opposing the motion is going to equal the coefficient of kinetic friction multiplied by the normal force and we can divide both sides by μ and solve for F N. So F N is the friction force which we assume is the same as the applied force because she's going to be moving at a constant speed—we suppose. So the friction force and the applied force are the same meaning that the friction force is going to be the 300 newtons that is the same as the applied force. Okay! So we have 300 newtons divided by coefficient of kinetic friction of 0.3 which is 1000 newtons is the total normal force. In part (b), we are asked to suppose that the steel is oiled and what friction force, and therefore, applied force would be needed then? And the answer is 0.03 because that's the coefficient of kinetic friction for oiled steel on steel multiplied by the same 1000 newton normal force—which isn't going to change— and that is 30 newtons of friction.

Comments

since the normal force is calculated using the coefficient of friction, isnt part b circular reasoning because now the normal force would be different when using oil?

We know that whether a box is sitting on concrete or ice the normal force is the same. The only time it would change is if the box was pushed down or pulled up on by an external force.

It is however reasonable to think that by adding a thin layer of molecules (oil) between the surfaces of the piston and cylinder that the normal force would increase slightly but I believe we are to assume it's negligible.

Understand the setup of the problem but why use the kinematic coefficient of friction and not the static? The problem doesn’t state that the piston was in motion when the force was applied.

Hi dslangston, thank you for the question. I suppose strictly speaking there is some room for interpretation between kinetic vs static friction. From the phrase that the student will "insert a dry steel piston into a steel cylinder" I feel pretty confident picturing the insertion as a movement, in which case it's kinetic friction. Suppose the student holds the piston initially outside of the cylinder and then proceeds to insert it - in this case the piston is never at rest with respect to the cylinder and only kinetic friction will apply.
Hope this helps,
Shaun

Strictly speaking, wouldn't there be no movement if the exerted force on the piston is equal to the opposing frictional force? If so, does that mean the 300 N of exerted force here is actually ever so slightly greater than that?

Hi rrincones, good question. It's OK for the applied force to be exactly equal to the friction force while the piston is being inserted. Having balanced forces means there is no acceleration. In order to get the piston up to speed, the student must have exerted a force slightly greater than 300N for some brief moment when initially inserting, but then dropped the force back to 300N for the vast majority of the time.
All the best,
Shaun