Question
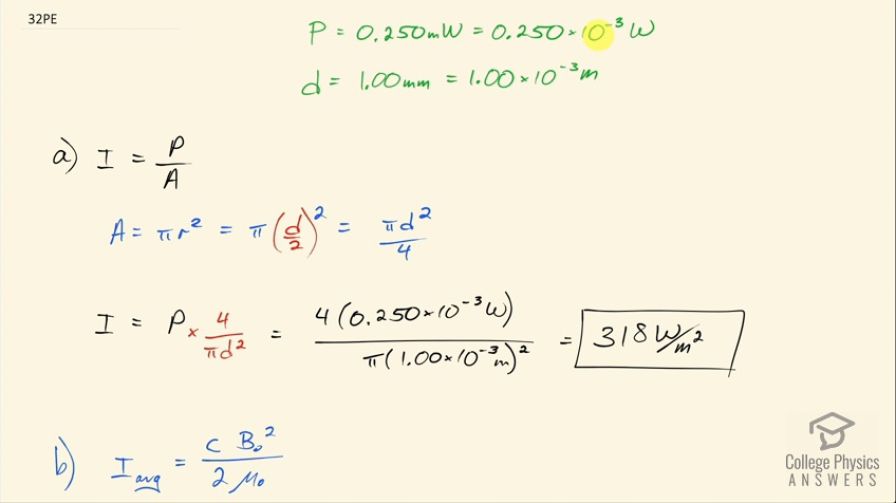
Assume the helium-neon lasers commonly used in student physics laboratories have power outputs of 0.250 mW. (a) If such a laser beam is projected onto a circular spot 1.00 mm in diameter, what is its intensity? (b) Find the peak magnetic field strength. (c) Find the peak electric field strength.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 24, Problem 32 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A helium neon laser has a power output of 0.250 milliwatts, which is 0.250 times 10 to the minus 3 watts and it casts light on a spot with a diameter of 1.00 millimeter, which is 1.00 times 10 to the minus 3 meters and the question in part (a) is what is the intensity of this light? And so we can divide the power output by the area over which that power is spread and that is the intensity. So the area is the area of the circular spot which is π times the radius of this spot squared but we are given the diameter so we divide that diameter by 2 and square that so we have π times diameter squared over 4— that's going to be our area— and now we are going power divided by this area and since it's a bit messy to have a fraction divided by a fraction, I am going to instead multiply power by the reciprocal of this area. So I am multiplying power by the flipped version of this which is 4 over πd squared. So the intensity is 4 times 0.250 times 10 to the minus 3 watts divided by π times 1.00 times 10 to the minus 3 meters squared which is 318 watts per square meter. Part (b) is asking for the peak magnetic field strength in this electromagnetic wave and we have this formula saying that intensity is the speed of light times the peak magnetic field strength squared divided by 2 times the permeability of free space. So we can rearrange this to solve for B naught squared by multiplying both sides by 2μ naught over c and this formula has intensity average here and that's what this is up here too... this is the average intensity. Okay! So we have B naught squared equals 2μ naughtI avg over c and then we take the square root of both sides to get the peak magnetic field strength. So that's square root of 2 times 4π times 10 to the minus 7 tesla meters per amp times 318.3099 watts per square meter— as we calculated it in part (a)— divided by the speed of light of 2.998 times 10 to the 8 meters per second and that's magnetic field strength of 1.63 times 10 to the minus 6 tesla. Part (c) asks for the peak electric field strength in this electromagnetic wave and so we use this formula which says the average intensity is speed of light times permeability of free space times the peak electric field strength squared over 2 and we will rearrange this for E naught squared by multiplying both sides by 2 over cε naught and then we have this line here and we take the square root of both sides to solve for the peak electric field strength, which is the square root of 2 times the intensity divided by speed of light times permeability of free space. So then we have... or permittivity I should say of free space there. So square root of 2 times 318.3099 watts per square meter divided by speed of light times permittivity of free space and this works out to 490 newtons per coulomb or you could also write this as volts per meter.

