OpenStax College Physics for AP® Courses, Chapter 24, Problem 38 (Problems & Exercises)

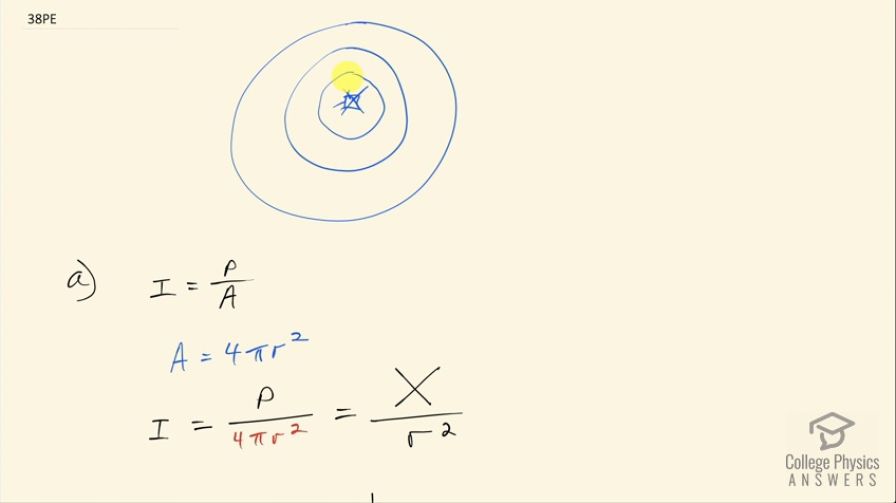
This is College Physics Answers with Shaun Dychko. We suppose that electromagnetic radiation is radiating uniformly in all directions so it's going to be spread over a sphere with some surface area at each distance from the source here and so the intensity will be decreasing as you get further from the source because the same given power output of the source is going to be distributed over a larger and larger area as you go further from the source— it's gonna be distributed over a larger sphere as you go away from the source. So this distance is the distance r here and we want to show that intensity is inversely proportional to r squared. So intensity is power divided by the area over which that power is distributed and the area in this case has formula 4πr squared since that's the area of the sphere. So we replace A with 4πr squared here and we can see then that intensity equals some stuff which I call letter X here divided by r squared so X is P over 4π and I am writing that just to illustrate that we have a factor divided by r squared and this means that intensity is proportional to 1 over r squared when you can show that it's equal to something times 1 over r squared—as we have here— X times 1 over r squared that means that the intensity is proportional to 1 over r squared. Okay! Part (b) asks us to show that the magnitudes of the electric and magnetic fields are inversely proportional to r to the power of 1. So let's talk about the peak electric field first and so we have this formula for intensity: it's the speed of light times the permittivity of free space times the electric field strength squared over 2 and the intensity is power divided by 4πr squared and we can solve this for E naught squared by multiplying both sides by 2 over cε naught and we have then that the electric field strength squared is 2 times power over c times permittivity of free space times 4π times r squared. Then take the square root of both sides and we have E naught equals some stuff multiplied by 1 over r so that means it's proportional to 1 over r where this stuff can be encapsulated in a single factor X and it is square root 2 times power over cε naught4π. Okay! Then we are gonna show that the magnetic field strength is also proportional to 1 over r starting with this formula for intensity: c times magnetic field strength at its peak squared over 2 times permeability of free space and that equals the intensity formula that we found before and then solve for B naught squared 2μ naught over c multiplying both sides by that and we get B naught squared is all this and then take the square root of both sides and you get B naught then is the square root of all this times 1 over r. So that means we have shown that B naught equals some factor times 1 over r which is to say that B naught is proportional to 1 over r.