OpenStax College Physics for AP® Courses, Chapter 24, Problem 44 (Problems & Exercises)

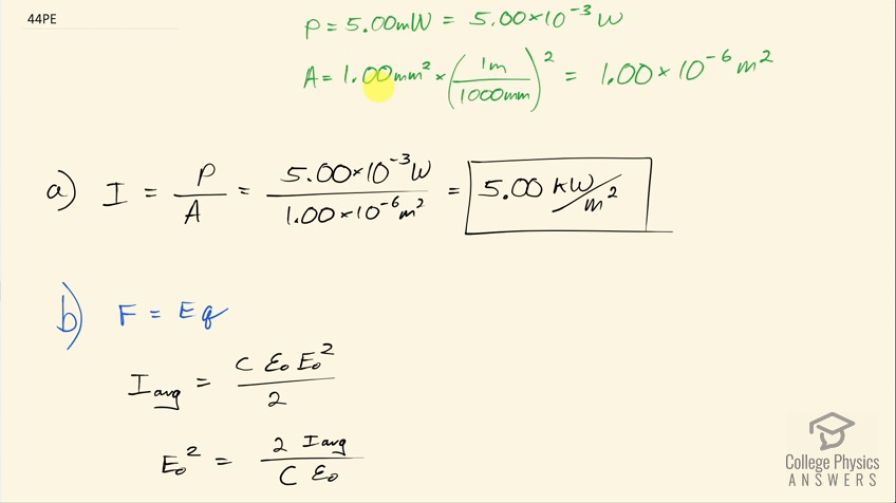
This is College Physics Answers with Shaun Dychko. A 5.00 milliwatt laser is projected on a 1.00 square millimeter of area and the question in part (a) is what is the intensity of this light? So we take the power divided by the area to get intensity so that's 5.00 times 10 to the minus 3 watts divided by 1.00 times 10 to the minus 6 square meters after converting these square millimeters into square meters by multiplying by 1.00 meter for every 1000 millimeters twice, this works out to 5.00 kilowatts per square meter. Part (b) is asking suppose a 2.00 nanocoulomb static charge was in the beam, what's the maximum electric force it would experience? So the force will equal the electric field that it experiences times its charge and the electric field is the electric field of the electromagnetic radiation. So we need to figure out what that is first and we know its intensity based on part (a) and we have another expression for intensity in terms of electric field strength at its peak so let's solve this for E naught eventually. So first we'll solve for E naught squared by multiplying both sides by 2 divided by speed of light times permittivity of free space and we get that the peak electric field strength squared is 2 times intensity divided by speed of light times permittivity of free space and then take the square root of both sides to solve for E naught. So E naught gets substituted in for the electric field here in this formula for force. So that's square root of 2 times intensity divided by speed of light times permittivity and then we multiply that by the charge. So the force it will experience then is square root of 2 times 5000 watts per square meter divided by 2.998 times 10 to the 8 meters per second—speed of light—times 8.854 times 10 to the minus 12 coulomb squared per newton meters squared— permittivity of free space— times 2.00 nanocoulombs written as 2.00 times 10 to the minus 19 coulombs and we get 3.88 times 10 to the minus 6 newtons. And then part (c) says well what about the force due to the magnetic field in this electromagnetic wave? So we use this formula which is the charge times the speed that the charge is moving times the magnetic field strength to get the force and we assume that the magnetic field and direction of the velocity are perpendicular to get the maximum possible force. So we take this formula here for intensity in terms of magnetic field strength and solve for B naught. We'll multiply both sides by 2 times permeability of free space divided by speed of light and then we have this expression here, we take the square root of both sides and we get then that the magnetic field strength is square root of 2 times permeability of free space times intensity divided by speed of light and we substitute that in here and multiply by the charge and also by its velocity. So that's 2.00 times 10 to the minus 9 coulombs times 400 meters per second we are told is its speed times square root of 2 times 4π times 10 to the minus 7 tesla meters per amp times 5000 watts per square meter divided by speed of light and we have 5.18 times 10 to the minus 12 newtons is the force due to the magnetic field of this radiation on the 2.00 nanocoulomb charge.