Question
Calculate the displacement and velocity at times of (a) 0.500, (b) 1.00, (c) 1.50, and (d) 2.00 s for a ball thrown straight up with an initial velocity of 15.0 m/s. Take the point of release to be .
Final Answer
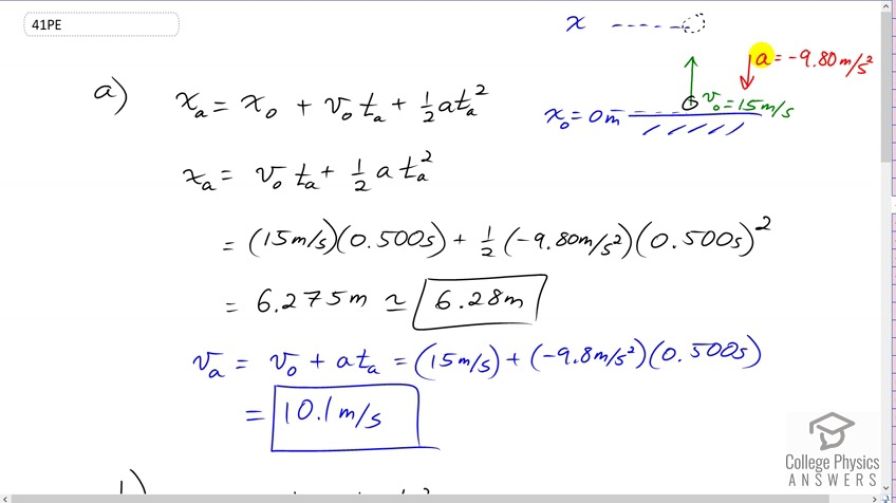
a) ,
b) ,
c) ,
d) ,
Solution video
OpenStax College Physics, Chapter 2, Problem 41 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko A ball is thrown straight up with an initial velocity of 15 meters per second. At all times, it has an acceleration due to gravity of negative 9.80 meters per second squared. It's negative because the acceleration is directed downwards and we're taking positive to be in the upper direction. The initial position is 0 meters and the final position is something we are going to calculate in each part of this question, given a different amount of time in each part. And we'll also find out what the velocity is at this final position. So, in part A, I have x subscript a to mean the position for part A of the question, equals the initial position x naught plus the initial velocity v naught multiplied by the time that has elapsed in Part A. So, I put a subscript a on the t here, plus one-half a ta squared. And x naught is 0. So, we're not going to see that variable anymore and we'll just reduce the equation to v naught ta plus one-half a ta squared. So, that's 15 meters per second times .5 seconds for part A plus one-half times negative 9.8 meters per second squared acceleration times .5 seconds squared, and this gives 6.28 meters will be the final position for Part A. To calculate the final velocity for Part A, that's going to be the initial velocity plus acceleration times the time elapsed for part A. And, that's 15 meters per second plus negative 9.8 meters per second squared times .5 seconds, giving 10.1 meters per second. And that's positive and so, it means the ball is still going in the upwards direction after half of a second. For Part B, we have the same formula as part A, but I've put subscripts b where they belong to label these as quantities for Part B of the question. So, we have b position. It's going to be the initial velocity, which does not need a separate b because it's the same initial velocity as it had in part A. So, it is called v naught. That's 15 meters per second. Multiplied by the time for Part B, which is a total of 1 second plus one-half times acceleration. Acceleration also does not have a subscript because it's the same in every part of the question. Acceleration due to gravity of negative 9.8 meters per second squared times by 1 second squared, which gives 10.1 meters. And then, the final velocity after 1 second will be 15 plus negative 9.8 meters per second squared times a second, which is 5.20 meters per second. So, we can see that it is slowing down as time progresses. So, .5 seconds, it was going 10.1 meters per second and now a full second has passed. And it's going at 5.2 meters per second, 5.2 being less than 10.1. And we'll see in part C that it is going slower still after 1.5 seconds. So, in part C, plugging in the numbers, we get 11.5 meters as the position and the speed is .3 meters per second. And then for Part D, we have all the quantities for part D here. The time being 2.0 seconds and otherwise initial velocity and acceleration being as the same as for the other parts of the question. And this is a position of 10.4 meters. So, notice that this position is less than the position was in part C. And so, you can see this formula does not give you distance traveled because the distance... by the time 2 seconds has passed, the distance traveled is greater than it was at one and a half seconds and yet this number is smaller. And that's because the position has been reduced since the ball has gone from... Let's go to the picture here. The ball has gone from here to its maximum position and is now back down. Some... Some ways to here. This is x - Part D. And up here is x - Part C. x- Part C is close to the very top because at the exact top, the velocity will be 0 and this is pretty close to 0. And so, at one and a half seconds, it's almost at the very peak of its trip. And then it comes back down. And we can see that it is moving down because the velocity for part D being 15 meters per second plus negative 9.8 meters per second squared times 2 seconds. The velocity is negative which means it's directed downwards in Part D. Negative 4.60 of meters per second.