Question
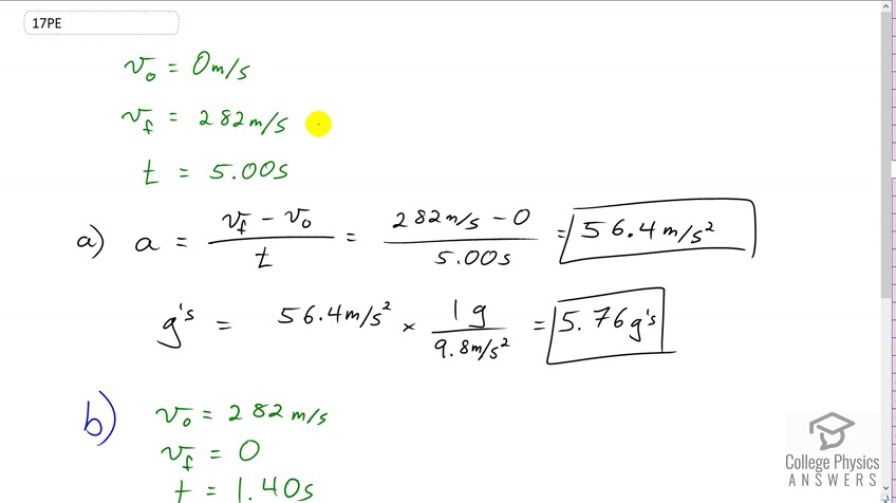
Dr. John Paul Stapp was U.S. Air Force officer who studied the effects of extreme deceleration on the human body. On December 10, 1954, Stapp rode a rocket sled, accelerating from rest to a top speed of 282 m/s (1015 km/h) in 5.00 s, and was brought jarringly back to rest in only 1.40 s! Calculate his (a) acceleration and (b) deceleration. Express each in multiples of gg (9.80 m/s2)(9.80 \textrm{ m/s}^2) by taking its ratio to the acceleration of gravity.
Question by OpenStax is licensed under CC BY 4.0
Final Answer

a) 56.4 m/s256.4 \textrm{ m/s}^2 or 5.76 g’s5.76 \textrm{ g's}

b) −201 m/s2-201 \textrm{ m/s}^2 or 20.6 g’s20.6 \textrm{ g's}

Solution video

OpenStax College Physics, Chapter 2, Problem 17 (Problems & Exercises)

OpenStax College Physics, Chapter 2, Problem 17 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 2, Problem 17 (PE) calculator screenshot 1
  • OpenStax College Physics, Chapter 2, Problem 17 (PE) calculator screenshot 2
Video Transcript
This is College Physics Answers with Shaun Dychko The experiment by Dr. John Paul Stapp was actually on YouTube and you can watch footage of him going on his rocket sled and you can also see how bad he looks after he's done. It actually injures him a bit and damages his eyes and his retina and so on. So, yeah, kind of a crazy guy, but let's calculate his acceleration. He went from 0 to 282 meters per second in 5 seconds. And so, I start the question as most questions, I start by writing down the information we've been given and I'm writing down just the part that's relevant for part A to begin with. So, acceleration is final velocity minus initial velocity divided by time and that's 282 meters second minus 0 divided by 5. That makes 56.4 meters per second squared. To calculate the number of gs or how many Earths' gravity this is equivalent to, we multiply that acceleration by 1 g for every 9.8 meters per second squared. And then, the meter per second squareds cancel and we are left with gs and that would be 5.76 gs. And then, after he is at his top speed, he decelerates to 1. ... in 1.4 seconds. And... Put a negative here actually. I just noticed this is missing. Acceleration is the final speed of 0 because he comes to a stop, minus the 282 meters per second he was going initially, divided by 1.4 seconds and that makes a negative 201 meters per second squared. And this negative means that the acceleration is directed in the negative direction. And it doesn't necessarily mean that he is slowing down by the way, although in this case, it does, but it doesn't always mean that. It just means it's in the negative direction. So, we have assumed that, supposed to the right as positive, and... and the velocity is that direction. And this negative means that the acceleration is in the opposite direction to the velocity. Well, at least, yeah. I suppose it's good enough. And then, gs divided by 9.8 and you get 20.6 gs on the deceleration.

Comments

Hi Andrea,
Thank you for the question. It wouldn't be wrong to say −20.6 g’s-20.6\textrm{ g's}, and I can see how one could argue that it's better. Nevertheless, it's tradition, I suppose, that when expressing an acceleration in terms of g's that it's expressed as a magnitude.
All the best,
Shaun