Question
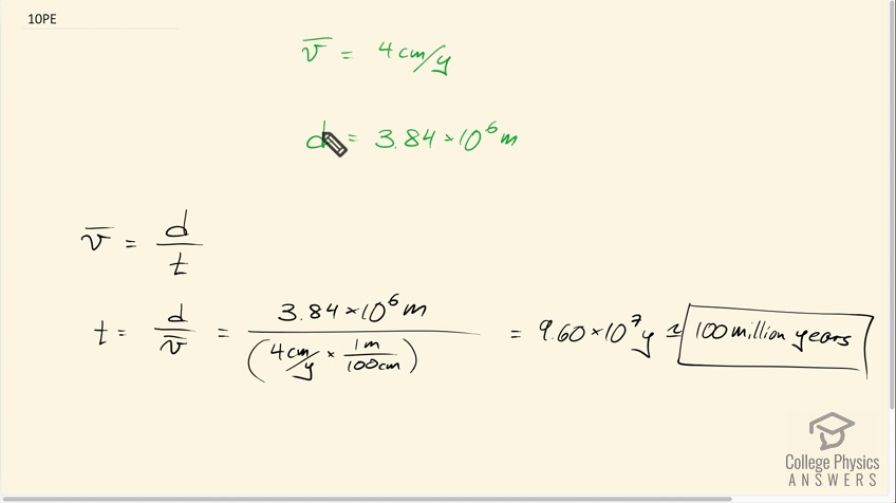
Tidal friction is slowing the rotation of the Earth. As a result, the orbit of the Moon is increasing in radius at a rate of approximately 4 cm/year. Assuming this to be a constant rate, how many years will pass before the radius of the Moon's orbit increases by (1%)?
Final Answer
100 million years.
Solution video
OpenStax College Physics, Chapter 2, Problem 10 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The radius of the Moon's orbit around Earth is increasing by 4 centimeters every year and the question is how long will it take for this increase to add up to 3.84 times 10 to the 6 meters which represents 1 percent of its current radius. So the average velocity of the change in its radius is going to be the displacement divided by time. So here's the Earth here in the center and we have this Moon orbiting around it and the Moon has this sort of radial velocity that is represented by this velocity with which it is increasing its radius and so it's gonna cover this much distance or this increase in radius in some amount of time that we have to calculate. So we'll rearrange this to solve for t by multiplying both sides by t and dividing both sides by the average velocity and we end up with time is displacement over average velocity. So that's 3.84 times 10 to the 6 meters and we are gonna divide by the velocity but being careful to convert the units so that we have meters on the bottom to cancel with the meters that are on top and our time unit in the end is going to be years because we have years in the bottom here in this velocity. So we have 3.84 times 10 to the 6 meters divided by 4 centimeters per year times 1 meter for every 100 centimeters and these centimeters cancel here leaving us with meters per year on the bottom. This works out to 9.60 times 10 to the 7 years but this is very approximate average velocity and with only one significant figure and so we should round this to one significant figure and this works out to 100 million years.
