Question
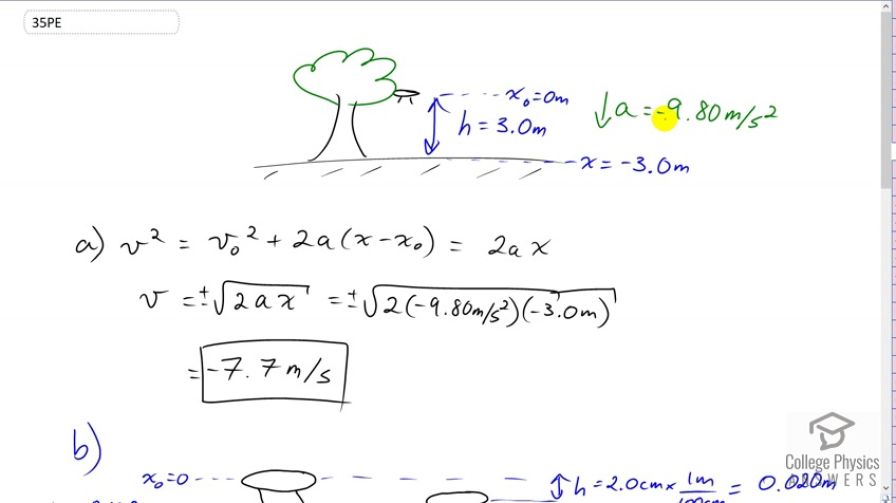
Consider a grey squirrel falling out of a tree to the ground. (a) If we ignore air resistance in this case (only for the sake of this problem), determine a squirrel's velocity just before hitting the ground, assuming it fell from a height of 3.0 m. (b) If the squirrel stops in a distance of 2.0 cm through bending its limbs, compare its deceleration with that of the airman in the previous problem.
Final Answer
a)
b) , which is 3 times the acceleration of pilots landing without parachutes.
Solution video
OpenStax College Physics, Chapter 2, Problem 35 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko When the squirrel jumps from the tree, it falls a height of 3.0 meters and the acceleration is that due to gravity, which is negative 9.80 meters per second squared. And so, I've labeled the initial position to be 0 meters and that means the final position is negative 3.0 meters, taking down to be the negative direction. And... We have to find the final speed by saying final speed squared equals initial speed squared plus 2 times acceleration times displacement. Now, the initial velocity is 0 and the initial position is 0. And so, this equation reduces to 2ax. And so, we have the square root of both sides It's what we will do to solve for v, and when you take the square root of both sides of an equation, you're not sure whether the right side should be positive or negative. It could be either. And so, we look at the physics of the situation to determine which one to take. And it turns out we're going to take the negative because we can see that the squirrel will be going in the downward direction which we've decided in our coordinate system is negative. So, we have final velocity as the negative of the square root of 2 times negative 9.8 meters per second squared times negative 3.0 meters, which gives a negative 7.7 meters per second. And then in part B, imagine that the squirrel initially touches the ground with their legs basically outstretched. And then... And then the squirrel bends its legs in order to cushion the landing, in order to make the deceleration occur over some distance. And so, it doesn't get injured. And we have to figure out what is the acceleration here and the height over which it bends its legs is 2 centimeters. And so, let's break that information down in our initial picture here, but convert it into MKS units - meters, kilograms and seconds are the units we always want. So, we'll convert this 2 centimeters by multiplying by 1 meter for every 100 centimeters, giving us .02 meters. The initial velocity here is negative 7.668 meters per second and I looked at my calculator and used an unrounded version of this answer from part A. Normally, we don't like using answers from one part in the next part, but in this question, it's unavoidable because we need some more information here to figure out the answer. So, because otherwise, all we know is the initial and final position. and... and you know, with only that information, there's nothing we can do. So, we also need to know what the initial and final speeds are. Okay. So... we have the same formulas we used in part A. It's going to be useful again. And we... We do some algebra on it by subtracting the initial squared from both sides. And then switch the sides around, so that we have 2a times x minus x naught equals v f squared minus v naught squared. And then, divide both sides by 2 times the displacement. This gives acceleration is final velocity minus initial velocity divided by 2 times the final position minus the initial position. So, that's 0 minus the negative 7.668 meters per second squared and the square applies to the negative here. So, this negative velocity 1 squared becomes positive, but there's a negative here. And so, this numerator will be negative in the end. And... And then, in the denominator, we have 2 times 0 for... So, I just switched the order of these numbers around here because I realize that the final position is negative .02 meters here. Final position... and the initial position is 0. And so, I have a negative .02 meters final position minus initial of zero. So, working this out, you get positive 1.5 times 10 to the 3 meters per second squared and the answer is positive because the initial velocity is negative. This acceleration is in the opposite direction to the initial velocity. And so, it should have the opposite sign. And that's why it is positive, directed upwards, and the acceleration of pilots jumping from their World War II fighter planes without a parachute, when they, you know, crash through branches and snow banks, their acceleration was negative 486 meters per second squared. And the... And to compare to the acceleration of the squirrel here, we can divide the 2 numbers. And so, we have 1.47 meters per second squared negative divided by negative 486 meters per second squared, which gives a factor of 3. So, the scroll acceleration is about a factor of 3 times more than the pilot acceleration.
