Question
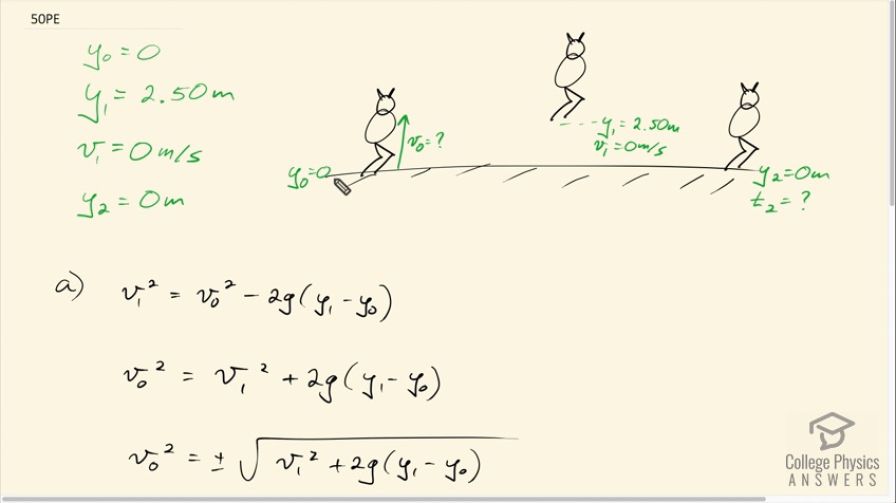
A kangaroo can jump over an object 2.50 m high. (a) Calculate its vertical speed when it leaves the ground. (b) How long is it in the air?
Final Answer
Solution video
OpenStax College Physics, Chapter 2, Problem 50 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This kangaroo leaps up in to the air with some initial velocity that we have to find, v naught, and they start at a height of zero say and they reach a maximum height of 2.50 meters and at that point, the velocity will be zero as it always is at the maximum height of a object, you know, that's free falling so to speak; I mean it's going upwards but it's being acted upon just by gravity and then it will return back to the ground at some time t 2. So part (b) we'll have to figure out t 2 and for part (a), we need to use this information here with the maximum height to determine what v naught is. So the velocity at the maximum height, v 1 squared, equals v naught squared minus 2 times acceleration due to gravity times the change in position and this is equation 77 from chapter 2. And we can rearrange this to solve for v naught by adding this term to both sides and then switching the sides around and we get v naught squared equals v 1 squared plus 2g times y 1 minus y naught. and then we take the square root of both sides and technically, it's the plus or minus square root but we can already tell from our picture that our initial velocity is positive so we are gonna be taking the positive route here and this should be v naught. Okay. So we have v naught is plus or minus the square root of 0 meters per second—that's the velocity at the maximum height—squared plus 2 times 9.8 meters per second squared times the maximum height of 2.50 meters minus the initial height of 0 giving us 7.00 meters per second must be the launch velocity of the kangaroo. In part (b), we want to know what is the total time that they spend in the air and so this equation here which is equation 76 from chapter 2 tells us that the final position equals the initial position plus the initial velocity multiplied by the time minus one-half times acceleration due to gravity times the time squared. Now y 2 is this position here which is back to the ground level which is 0 meters and it started at 0 meters and add to that the initial velocity that we found already times t 2 minus one-half times gt 2 squared. Now we can divide everything by t 2 which gets rid of the t 2 factor there and then makes this t 2 to the power of 1 and then bring this term to the left side which makes it positive so that's positive one-half g times t 2 and then multiply both sides by 2 and divide both sides by g— maybe I can show more of that, maybe it can make more sense. So the intermediate step here is that we have positive one-half g times t 2 equals v naught that's left behind on the right hand side and then we multiply both sides by 2 over g and you end up with this line here. So t 2 is 2 times the initial velocity divided by acceleration due to gravity. So that's 2 times 7.00 meters per second divided by 9.80 meters per second squared which is 1.43 seconds.
