Question
In World War II, there were several reported cases of airmen who jumped from their flaming airplanes with no parachute to escape certain death. Some fell about 20,000 feet (6000 m), and some of them survived, with few life- threatening injuries. For these lucky pilots, the tree branches and snow drifts on the ground allowed their deceleration to be relatively small. If we assume that a pilot's speed upon impact was 123 mph (54 m/s), then what was his deceleration? Assume that the trees and snow stopped him over a distance of 3.0 m.
Question by OpenStax is licensed under CC BY 4.0
Final Answer

5×102 m/s25\times 10^{2}\textrm{ m/s}^2

Note: The calculator screenshot shows the acceleration is negative, but I should have surrounded the −54 m/s-54\textrm{ m/s} in brackets, in which case squaring it would have made it positive. We know the acceleration should be positive since it's reducing a downward velocity. It's in the opposite direction to the downward velocity, and therefore is directed up. Upward was defined, as usual, to be positive, which makes the acceleration positive.

Solution video

OpenStax College Physics, Chapter 2, Problem 34 (Problems & Exercises)

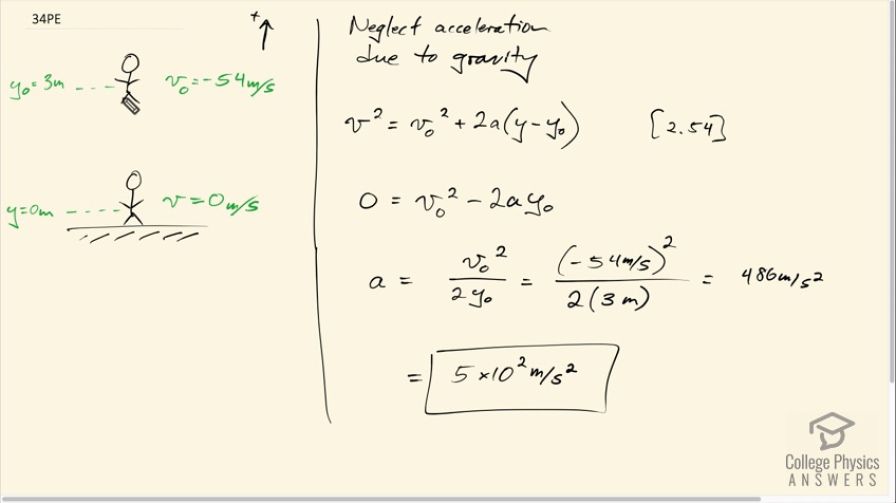
OpenStax College Physics, Chapter 2, Problem 34 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 2, Problem 34 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. A fighter pilot in World War 2 leapt out of their burning fighter plane and reached some tress or maybe a snow bank that was 3 meters above the ground with some initial velocity of negative 54 meters per second so the velocity is downwards and that's why it's negative since we have taken upwards to be the positive direction. The branches and the snow bank or whatever it is that they are hitting slows them down to the point where they reach zero velocity by the time they reach the ground. So we are going to figure out what their acceleration is due to these branches and we use equation [54] to figure it out; the final velocity squared equals the initial velocity squared plus 2 times the acceleration they experience multiplied by their change in position. And we are neglecting gravity here; gravity is accelerating them downwards but the branches are much more significantly applying a force upwards and so their acceleration is going to be very much upwards which makes sense because their velocity is, you know, downwards and it's slowing down so there must be acceleration upwards and the force applied by the branches is going to be very much more than the force downwards due to gravity and so the force due to gravity will be insignificant and we can neglect it. So the final velocity is zero and the initial velocity we are told and that's minus 2 times acceleration multiplied by the initial position; we substituted zero for the final position, y, here as well and then this minus here is the result of multiplying 2a by this negative y naught. So we solve this for a; we add 2ay naught to both sides and then divide both sides by 2y naught and we get acceleration is the initial velocity squared divided by 2 times the initial position. So that's negative 54 meters per second squared divided by 2 times the beginning of the trees which is at the 3 meters and that equals 486 meters per second squared. This is a rather imprecise calculation we are doing here and we have only one significant figure in our initial position so we'll round this to one significant figure and we'll call it 5 times 10 to the 2 meters per second squared; this is a positive acceleration, the acceleration is directed upwards and there we go.

Comments

The answer is wrong, its a negative.

Hello, thank you for the comment. The calculator screenshot shows a negative answer, but the mistake is in the screenshot. I should have surrounded the −54 m/s-54 \textrm{ m/s} in brackets, in which case squaring it would have made a positive. The acceleration has to be positive since it's directed upward, and upward was defined (as usual) to be positive. We know the acceleration is positive since it reduces downward velocity. It's in the opposite direction to the downward velocity, in other words.

why we are not putting the intital distace 6000m intead of putting 3m ?

Hello, thank you for the question. This question asks us to calculate the deceleration of the airmen. The deceleration occurs over a distance of 3m. Only the initial and finals velocities, and distance over which this change in velocity occurs, is important to calculate deceleration. The 6000m figure given is the distance over which they accelerated and then traveled at terminal velocity before impacting the trees/snow/ground.
Hope this helps,
Shaun

Hello, I thought when we consider deceleration the answer would always be negative.