(a) Calculate the rate of heat transfer by conduction through a window with an area of 1.00 m ^2 that is 0.750 cm thick, if its inner surface is at 22.0° C and its outer surface is at 35.0° C . (b) What is unreasonable about this result? (c) Which premise or assumption is responsible?

Name: OpenStax College Physics Solution, Chapter 14, Problem 78 (Problems and Exercises)
Uploaded: 2020-05-21T17:43:37-07:00
Duration: 106 s
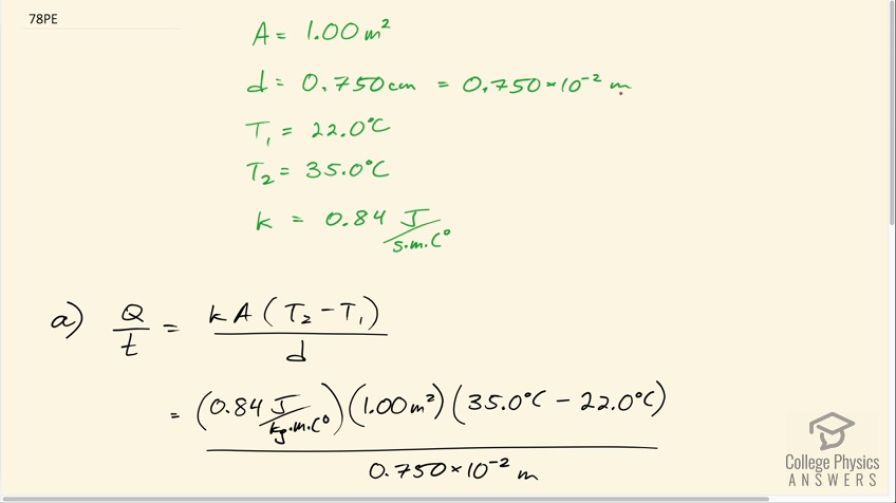
Description: (a) Calculate the rate of heat transfer by conduction through a window with an area of 1.00 m ^2 that is 0.750 cm thick, if its inner surface is at 22.0° C and its outer surface is at 35.0° C . (b) What is unreasonable about this result? (c) Which premise or assumption is responsible?

a) 1.5 x 10^{3} W b) This is too large. If windows lost heat at this rate, then it would be impossible for 1500W heaters to keep a room warm. c) The temperature difference between sides of the window must not be so great.

OpenStax College Physics for AP® Courses, Chapter 14, Problem 78 (Problems & Exercises)

In order to watch this solution you need to have a subscription.

Start free trial Log in

vote with a rating of votes with an average rating of .

This is College Physics Answers with Shaun Dychko. A window with an area of 1.00 square meter has a thickness of 0.750 centimeters which is 0.750 times 10 to the minus 2 meters and the temperature on the inside of it is 22.0 degrees Celsius and the temperature on the outside is 35.0 degrees Celsius and the thermal conductivity of glass is 0.840 joules per second per meter per Celsius degree. So what is the rate of heat transfer across this window due to conduction? That will be the thermal conductivity times the area times the difference in temperatures divided by the thickness. So that's 0.84 times 1.00 square meter times 35.0 degrees Celsius minus 22.0 degrees Celsius divided by 0.750 times 10 to the minus 2 meters and that's 1.5 times 10 to the 3 watts— that number is too large. If windows lost heat this fast then it would be impossible for a 1500 watt heater to keep a room warm. 1500 watts is the maximum power you can plug into a standard 15 amp service. So most plugs in North America are connected to a circuit breaker rated at 15 amps and 15 amps times 120 volts is approximately 1500 watts. I mean this is, you know, leaving a bit of room for error... this is the maximum wattage of heaters that are sold in North America. And these heaters do keep a room warm and so that contradicts this idea that windows could lose heat so fast. Okay! So that must mean the temperature difference between the sides of the window is not so great because everything else is reasonable; this thickness is reasonable, the area is reasonable but either this temperature outside is lower or this temperature inside is higher or some combination of the two.