Question
One half the rays from are absorbed by a 0.170-mm-thick lead shielding. Half of the rays that pass
through the first layer of lead are absorbed in a second layer of equal thickness. What thickness of lead will absorb all but one in 1000 of these rays?
Final Answer
Solution video
OpenStax College Physics, Chapter 32, Problem 12 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
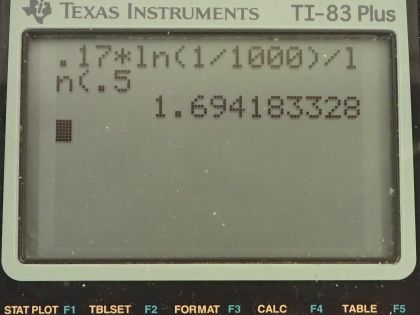
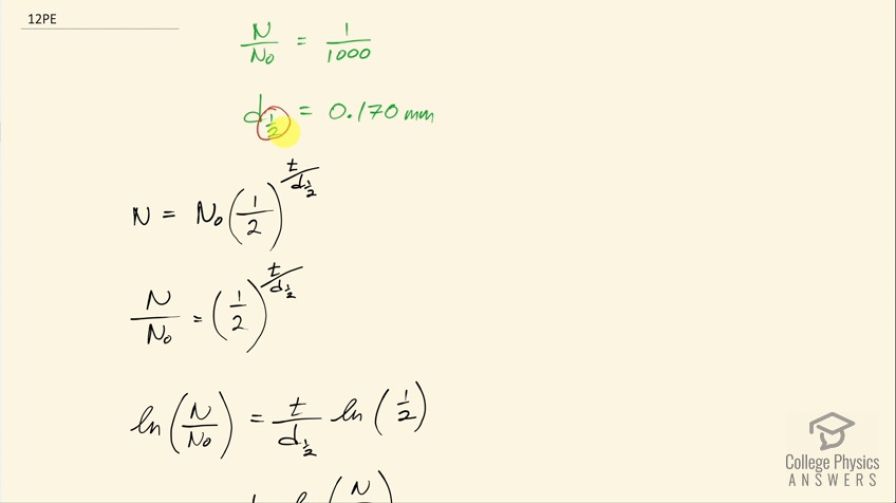
This is College Physics Answers with Shaun Dychko. We are told that a 0.170 millimeter thickness of lead blocks half of the gamma rays that are passing through it created by technetium-99 decay so the question is what total thickness is needed then to have 1 in a 1000 gamma rays pass through the total thickness? So we have one thickness here of width d subscript one-half and then another thickness here again d subscript one-half and these gamma rays are being emitted by some technetium-99 and half of them will get absorbed by this layer and then half of the ones that remain will get absorbed by the next layer and then so on and so on and then by the time you get to the end here, you have only one out of the original 1000 making it this far. Okay! So the total number that end up at the end... so this is N is one-one thousandth of what we had originally or you can say that the number that pass through is the original number that hit the beginning multiplied by one-half as many times as there are thicknesses of lead and that's what this fraction is. This fraction tells how many layers of 0.170 millimeters of lead do you have so this t is what we are solving for— this total thickness. Okay! So we can divide both sides by N naught and we get the total number that pass through divided by the original number that hit equals one-half to the power of total thickness divided by the thickness for absorbing half. This N over N naught is 1 over a thousand and we can take the natural logarithm of both sides here because we want to solve for this factor in the exponent and so taking natural logarithm of both sides we have ln of N over N naught on the left and on the right side, one of the logarithm rules is that when you take the log of something with an exponent, you can turn that exponent into a factor in front so we have t over d one-half times natural logarithm of a half on the right side. And then we multiply both sides by d one-half divided by natural log of 2 or natural log of a half I should say and then we solve for t that way. So t is the thickness that blocks half the gamma rays times natural logarithm of the fraction that penetrates divided by natural logarithm a half. So that's 0.170 millimeters times natural log of 1 over a 1000 divided by natural log of a half and that's 1.69 millimeters.