Question
A plumber at a nuclear power plant receives a whole-body dose of 30 mSv in 15 minutes while repairing a crucial valve. Find the radiation-induced yearly risk of death from cancer and the chance of genetic defect from this maximum allowable exposure.
Final Answer
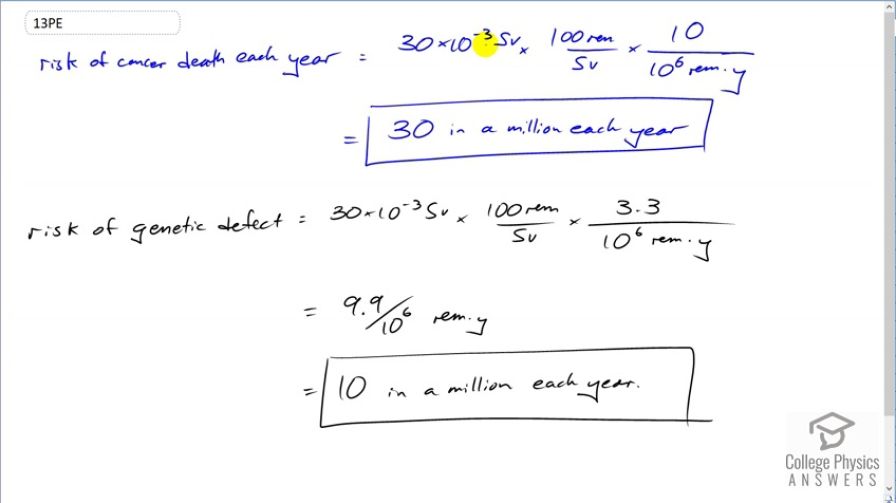
risk of cancer death each year = 30 in a million each year.
risk of genetic defect = 10 in a million each year.
Solution video
OpenStax College Physics, Chapter 32, Problem 13 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. The worker at this nuclear power plant repairs a crucial valve and is exposed to 30 millisieverts while doing that work. And the question is what is the risk of cancer death that this worker now has as a result of this exposure each year? Now it's not the exposure that happens each year, it's the risk of cancer death each year resulting from this single exposure. So 30 millisieverts is written as 30 times 10 to the minus 3 sieverts and we have to convert this into rems because we are told in the textbook that there's a risk of 10 in a million risk of cancer death each year per rem of exposure. So we multiply by 100 rems per sievert to get our exposure in rems then multiply by 10 in a million and we have 30 in a million is the risk each year that this worker will die from cancer as a result of this exposure. That's a pretty small risk; 30 in a million. Now the risk of genetic defect is 3.3 in a million per rem per year and so we have 30 millisieverts converted into rem multiplied by 3.3 divided by a million and this gives 9.9 or 10 in a million risk each year of genetic defect due to this exposure.