OpenStax College Physics, Chapter 32, Problem 41 (Problems & Exercises)

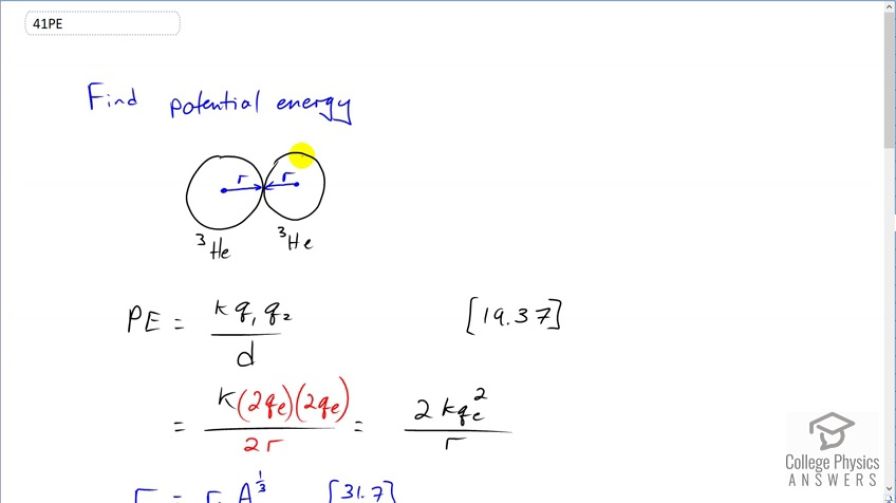
This is College Physics Answers with Shaun Dychko. We want to find the temperature of a gas such that two helium-3 nuclei will come just in contact with each other. And so we have this picture here where this is one nucleus just touching the other spherical nucleus. The distance between their centers will be 2 times the nuclear radii. Now there's gonna be a potential energy associated with this configuration due to the electrostatic repulsion between the protons and the nuclei. And so that potential energy will be coulomb's constant times the charge in the first nucleus times the charge in the second nucleus divided by the distance between the centers. So distance between the centers is 2r and the charge on each is 2 times the elementary charge because they have two protons in each nucleus. So that's a total of four q e on the top and 4 divided by 2 is 2. So we have this is the electrostatic potential of this of having the helium nuclei in these positions. So we can figure out what the radius of a helium nucleus is based on this equation [31.7] where we have r naught which is 1.2 femtometers multiplied by the mass number of the nucleus so in this case it's 3 and that's raised to the power of one-third. So we substitute that in for r and that's what's showin in red here. And then we'll consider the kinetic energy that this gas needs to have because kinetic energy is related to the gas's temperature which is what we want to find ultimately. And we know that the average kinetic energy needs to be half of this potential energy and this question tells us that. And so we can substitute the potential energy in here and then the 2's cancel and so the average kinetic energy is gonna be coulomb's constant times the elementary charge squared divided by r naught times the mass number to the power of one-third. And we also know from chapter 13, equation 55 that the average kinetic energy is 3 over 2 times Boltzmann's constant and I give the k a prime symbol here to distinguish it from the k here which is coulomb's constant. and now we are multiplying by the absolute temperature in Kelvin here. So we can equate this with this and we do that here and then solve for T by multiplying both sides by 2 over 3 k prime and we get this equation here for the temperature. So the gas temperature in order to bring two helium nuclei just barely touching each other it's 2 times coulomb's constant times the elementary charge squared divided by 3 times Boltzmann's constant times r naught times mass number to the power of one-third. Then we substitute in the numbers; 2 times coulomb's constant and here's the elementary charge squared divided by 3 times Boltzmann's constant times 1.2 femtometers and then the helium nuclei have three nucleons in them so the mass number is 3 to the power of one-third and we get 6.4 times 10 to the 9 Kelvin. Now that temperature exceeds the melting point of every known material and so controlled fusion is therefore really difficult because you have to find some technique to suspend the reaction away from any materials.