OpenStax College Physics, Chapter 7, Problem 22 (Problems & Exercises)

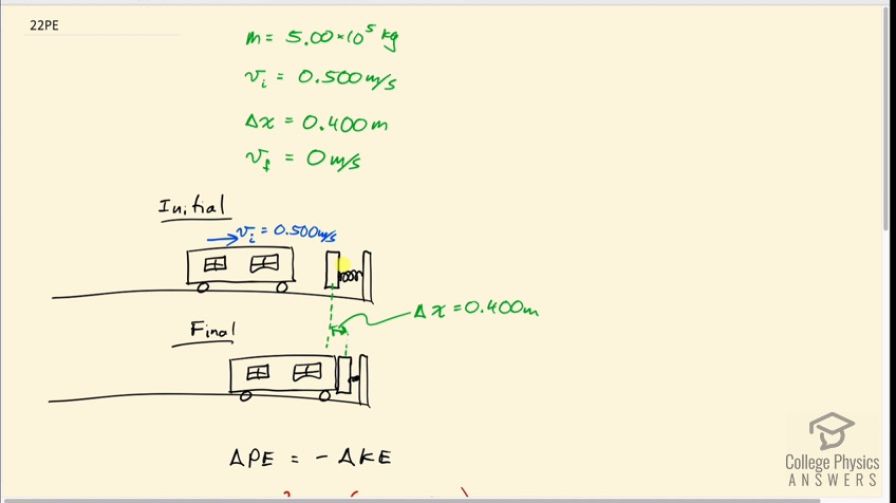
This is College Physics Answers with Shaun Dychko. A subway train car is initially going at a speed of 0.5 meters per second— let's suppose it's going to the right in this picture— and it hits a bumper which is connected by a spring to a wall here and it compresses that bumper—0.400 meters— before coming to a stop so at this point, this final moment, we have our v final is zero so it looses all of its kinetic energy. Now the only forces involved here are conservative forces that is the force exerted by this spring and so this loss in kinetic energy has to be made up for in a gain in potential energy somewhere else and so that is a gain in potential energy in the spring is going to equal the loss in kinetic energy of the subway car. So we write down all the information we know here: the subway car's mass is 5.00 times 10 to the 5 kilograms and it has an initial speed of 0.500 and it compresses the spring 0.400 meters. Okay! So conservation of energy says that the gain in potential energy due to compressing the spring is equal to the loss in the kinetic energy. And so that is one-half times Hooke's spring constant times the spring compression Δx squared and that equals the negative of the final kinetic energy minus the initial kinetic energy. Now the final kinetic energy is zero because it isn't moving in the end and so we have this negative and this negative making a positive initial kinetic energy on this right hand side. And then we solve for k by multiplying both sides by 2 over Δx squared. Actually I guess that's what I do in the next line isn't it so let's do this 2 times Δx squared down here and before that, we'll substitute one-half mv i squared in place of kinetic energy initial. Okay! So the 2's cancel here and the Δx squared's cancel there and we are left with k on the left side and on the right hand side, we have the 2's canceling and we have mv initial squared divided by Δx squared. So that is 5.00 times 10 to the 5 kilograms times 0.500 meters per second squared divided by 0.400 meters—spring compression— squared which is 7.81 times 10 to the 5. Now the units are kilograms per seconds squared is one way you could write it but that's a bit strange looking and so we can instead write this as newtons per meter because kilograms meters per second squared is the units for newtons— this could be written as N— and because this is, you know, mass times acceleration— Newton's second law, F equals ma— so the newtons comes from kilograms multiplied by acceleration and then we can make this kilograms per second squared by dividing the newtons by meters and then this meter in here will cancel and so it works out to being kilograms per seconds squared. Okay - newtons per meter is our unit for the spring constant.