OpenStax College Physics, Chapter 20, Problem 66 (Problems & Exercises)

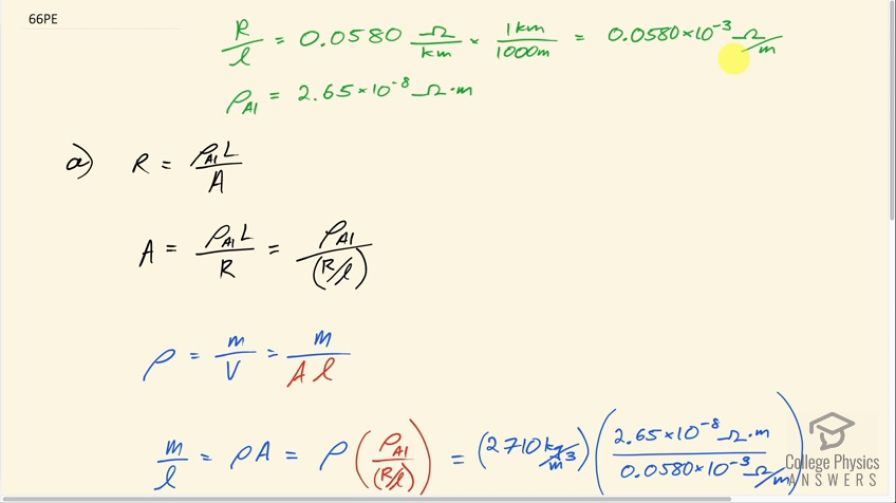
This is College Physics Answers with Shaun Dychko. The resistance per length of an aluminum wire is 0.0580 ohms per kilometer and we convert that into ohms per meter by multiplying by 1 kilometer for every 1000 meters and we always want to have our units in meters, kilograms and seconds. And the resistivity of aluminum we look up in ... table [20.1], 2.65 times 10 to the minus 8 ohm-meters and then we write down this formula for resistance; we are gonna find its mass per length by the way, that's the question here. So the resistance of the wire is the resistivity of aluminum multiplied by its length divided by its cross-sectional area and we can solve this for A by multiplying both sides by A over R and then we get an expression for the cross-sectional area and that's useful because when we talk about the mass density, which is what this ρ represents, it's a bit confusing with the symbols here because this ρ is the resistivity of aluminum and this ρ is the mass density of aluminum— kilograms per cubic meter, in other words. Okay! So density is mass per volume— that's the definition of density— and volume for a wire because it's a cylinder is going to be its cross-sectional area multiplied by its length and so we can see that we need to know what this area is in order to figure out the mass per length and that's what we did up here using this resistance formula, we found the cross-sectional area. Okay! So we can find mass per length by multiplying both sides by A and so mass per length then is the mass density multiplied by the cross-sectional area and then we substitute in the resistivity divided by the resistance per length. By the way, in case this from here to here is confusing... what we have here is L over R, which is the reciprocal of R over l; I mean it's a capital L here and a little l there but it's the same l, it's just traditional to have capital L in this formula. This is a reciprocal of that and so we can instead divide by (how do I say it?) we can divide by its reciprocal, which is what I have done here. So multiplying by L over R, which is what we are doing here is the same as dividing by the reciprocal of this factor so we can divide by R over L. Okay! (where are we now?) So we are down here, we have made the substitution and then we plug in numbers. So I had to look up in Google what the mass density of aluminum is— 2710 kilograms per cubic meter— times 2.65 times 10 to the minus 8 ohm-meters divided by 0.580 times 10 to the minus 3 ohms per meter and that gives 1.2382 kilograms per meter. But it's asking for the kilograms per kilometer so we multiply by 1000 meters for every kilometer and that's 1.24 times 10 to the 3 kilograms per kilometer. Now the mass per length of a copper wire would be the mass density of copper which is 8940 kilograms per cubic meter multiplied by the resistivity of copper— 1.72 times 10 to the minus 8 ohm-meters— divided by this same resistance per length we are told and this works out to 2.65 times 10 to the 3 kilograms per kilometer. So copper wire even though copper has a lower resistivity so it's a better conductor than aluminum, the mass density of copper is so much higher than that of aluminum that the kilograms per kilometer for the same resistance per kilometer will be higher for copper.