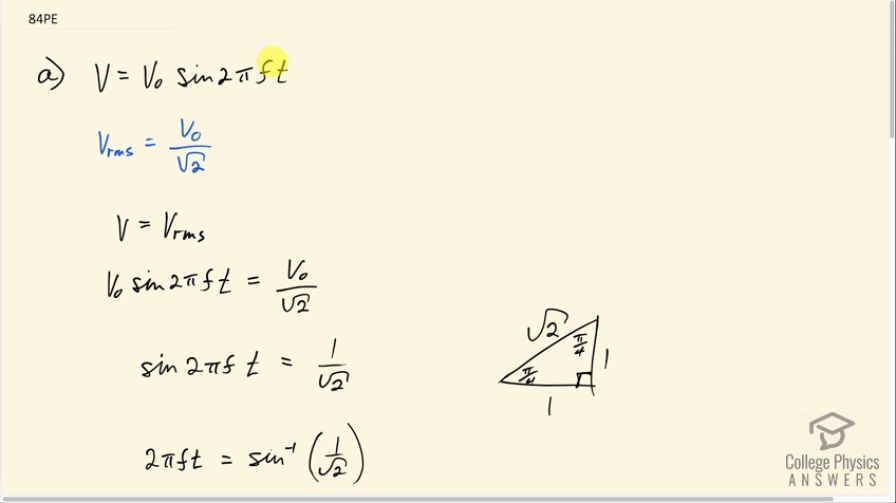
Question
(a) At what two times in the first period following does the instantaneous voltage in 60=Hz AC equal ? (b)
Final Answer
Solution video
OpenStax College Physics, Chapter 20, Problem 84 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
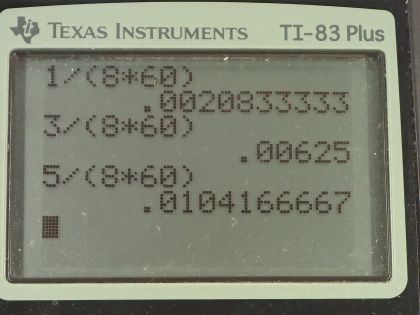
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The instantaneous voltage for alternating current is the peak voltage multiplied by sin of 2π times the frequency of the alternating current multiplied by time and the V rms is this peak voltage divided by square root 2 and we are told that the instantaneous voltage is going to equal the V rms and the question is at what times does this happen... which of the first two times it happens after time zero? So we have V naught sin 2πft, which is what the instantaneous voltage is, equals the V rms, which is V naught over root 2 and so we are solving this for t. So first we divide both sides by V naught and then we get sin 2πft equals 1 over root 2 then take the inverse sin of both sides so the inverse sin of sin is the argument of the sines so we have 2πft in the left; on the right hand side, we have inverse sin of 1 over square root 2. Now 1 over square root 2 comes from one of our special triangles which has legs of equal length and then the hypotenuse is square root 2 and the angles are π over 4 and π over 4 here and this is 90.0 degrees of course, this is 45.0 degrees but we are gonna write π over 4 in place of inverse sin of 1 over root 2 because the sin of this angle is what gives us 1 over root 2. So now we are looking for... trigonometric equations have an infinite number of solutions because since the sin function is periodic, it keeps passing the same Y value over and over and over again and the Y value we are interested in is 1 over root 2, whatever that number is, and it occurs here and then it occurs here and the question is what is the value on the x-axis when that happens? And so it's π over 4 in the first case and then over here, it's going to be 3π over 4 the second time this same Y value occurs and we know that because if you consider this this picture here, the unit circle, and this reference angle is π over 4— this is the angle between the terminal arm and the x-axis— we get the same reference angle at this standard position angle. So this reference angle here is also π over 4, the y-coordinate is also 1 over root 2 and this standard position angle is π minus the reference angle so π minus π over 4 which is 3π over 4. So that's another way of saying that the second solution for this trigonometric equation is 3π over 4. I mean that's... that's the solution to, you know, inverse sin of 1 over root 2 is 3π over 4. Okay! And so then we carry on to figure out what t is. So we divide both sides by 2πf and we end up with the π's canceling and we have 1 over 8 times f is the time then and that's 1 over 8 times 60 hertz, which is 2.1 milliseconds and then on this other solution here, we have time is gonna be 3 over 8f so that's 3 over 8 times 60 hertz, which is 6.3 milliseconds. So the two first times at which the instantaneous voltage equals the rms voltage, after t equals zero, is 2.1 milliseconds and 6.3 milliseconds. And then we say that the instantaneous voltage in part (b) is the negative of the V rms and so we have this instantaneous voltage formula equals negative V naught over square root 2 so the negative of the V rms and then dividing both sides by V naught gives us sin 2πft equals negative 1 over root 2 and taking the inverse sin again on both sides, we are left with 2πft on the left and on the right hand side, we have inverse sin of negative 1 over root 2. So we take the positive of this argument, which is 1 over root 2, take the inverse sin of that and our answer is π over 4 so that's our reference angle and then we need to look at which quadrant we have a negative sign in. So this is a pattern that I remember by the word "CAST" and you start in quadrant four and move counterclockwise following each of these letters. So starting with C, you go to A, S and T and these are the quadrants in which the trig functions are positive. So cosine is positive here, all the trig functions are positive in quadrant one the sin function is the only one positive in this quadrant and the tangent function is positive in this quadrant. So the two quadrants in which sin is negative are these two bottom quadrants so here and here and I have drawn these terminal arms such that the reference angle or in other words, the small positive angle between the terminal arm and the x-axis is π over 4 in which case, the standard position angle meaning we start from the positive x-axis and move counterclockwise is gonna be half of a circle, which is π, plus this π over 4 and π plus π over 4 is 4π over 4 plus π over 4 which is 5π over 4 and that's where this comes from. And then we have to find the standard position angle for this solution. And so that's going to be 2π, which will be a full circle, minus this reference angle which is π over 4. So this little bit here, this is π over 4, and we have 2π minus π over 4 which is 8π over 4 minus π over 4 which is 7π over 4. So now we divide both sides by 2π times frequency and we get the time is 5 over 8 times frequency, you know, it's 5 over 8 times 60 which is 10 milliseconds or we have t equals 7 over 8 times 60 hertz which is 15 milliseconds. And so the two initial times after t equals zero when the instantaneous voltage is the negative of the V rms, is 10 milliseconds and 15 milliseconds.